Acid–base balance disorders can be found in a primary or secondary form in patients with a disease process such as Diabetes Mellitus or acute renal failure, among others. The objective of this article is to explain and guide the correlationship between the clinical findings in the patient and the parameters of arterial blood gases in a simple and precise manner, in order to make the correct acid–base balance diagnosis and adequate therapeutic interventions. A non-systematic review of the scientific literature was conducted through a search in the PubMed, Science Direct, Scopus, and OvidSP databases. The conclusion was that base excess or deficit in arterial blood gases is a useful tool which along with the clinical history, pH, and partial pressure of CO2, provides an accurate estimate of the metabolic component of the acid–base balance.
Las alteraciones del Equilibrio Ácido-Base se pueden presentar en pacientes de forma primaria o secundaria a un proceso patológico como la Diabetes Mellitus o la falla renal entre otros. El objetivo es explicar y orientar la correlación clínica del paciente con los parámetros de los gases arteriales de manera sencilla y precisa, para realizar un diagnóstico de las alteraciones del Equilibrio Ácido-Base correcto, que permita efectuar intervenciones terapéuticas adecuadas y oportunas. Se realizó una revisión no sistemática de la literatura científica en la cual se consultaron las siguientes bases de datos: PubMed, ScienceDirect, Scopus y OvidSP en busca de artículos relevantes. Se concluyó que el exceso o déficit de base es una herramienta útil de los gases arteriales, que aunada a la historia clínica, el pH y la presión parcial de CO2 estima de forma muy precisa el componente metabólico del Equilibrio Ácido-Base.
Over time, researchers like Henderson, Hasselbalch, Stewart, Siggaard and Andersen, among others have contributed to the basic understanding of acid–base equilibrium. However, discussions are still ongoing regarding which should be the approach in clinical practice: a simple, reproducible approach or a complex approach with multiple laboratory parameters? Considering that, in the end, the clinical approach to the patient has diagnostic, prognostic and therapeutic implications, a fast accurate process is imperative1–7.
MethodologyA non-systematic review of the literature was conducted in the PubMed, ScienceDirect, Scopus and OvidSP databases. The following MESH terms were used for the advanced search: Acid-Base, Equilibrium OR Acid-Base imbalance OR Acidosis OR Alkalosis OR Hydrogen-Ion Concentration, included in the title, the abstract or the key words. The search was restricted to articles published after 2002.
The articles were then selected according to the title and the abstract and sorted by topics considered relevant by the authors for detailed review, including history, pathophysiology, consequences, divergences, clinical approach (according to various authors), diagnosis, prognosis and management.
Physiology in the Henderson and Hasselbalch traditional approachFree hydrogen ions (H+) are found in arterial blood at a concentration between 35 and 45nmol/L, which is the same as maintaining a pH between 7.45 and 7.35; pH is defined as the negative logarithm (base 10) of hydrogen ion concentration in blood8-10. Hydrogen ion (H+) concentration is one of the most important parameters in acid–base equilibrium and depends on the interactions between partial pressure of carbon dioxide (PaCO2), bicarbonate ion (HCO3−) plasma concentration, constant dissociation of carbonic acid, and carbon dioxide solubility, as determined by the Henderson and Hasselbalch equation. Carbon dioxide (CO2) reacts with water in a reversible way to form carbonic acid, which later dissociates into HCO3−+H+ (CO2+H2O↔H2CO3↔H++HCO3−). This reaction is catalysed by carbonic anhydrase, an enzyme found in red blood cells, nephrons, gut, pancreas, striated muscle, and lung capillary endothelia7,8,10,11.
Compensatory mechanismsIn order to maintain the acid–base balance in extracellular fluid, changes are compensated by:
- (1)
The respiratory system, which clears or retains CO2 through alveolar ventilation changes (hyperventilation or hypoventilation, respectively, in response to changes sensed by the chemoreceptors), leading to changes in PaCO2. Because of its low molecular weight and high solubility, CO2 crosses easily between the different membranes and biological compartments, altering [H+]4–6,9,12–16.
- (2)
The renal system, through the proximal tubule, which increases or lowers H+ secretion (acid) and resorbs close to 80% of filtered HCO3− 16% into the large ascending segment and the distal convoluted tubule, and the remaining 4% into the collecting tubule. But new bicarbonate is also produced through two mechanisms: (1) From glutamine (2/1) by deamination in the proximal tubule, resulting in alpha-ketoglutarate which is metabolised with CO2 and H2O to form HCO3−, while ammonium (NH4+) dissociates into ammonia (NH3) for transport to the tubular lumen. (2) From phosphates in the form of neutral salts filtered by the glomerulus that bind to H+ in the lumen, generating HCO3− in the cells of the proximal and distal tubules and the collecting duct in a 1:1 ratio, although they represent only a small fraction (titratable acidity). Bicarbonate then becomes the main non-respiratory metabolic control factor in acid–base equilibrium4–6,9,12–16.
Acute changes in blood pH induce regulatory effects on protein and enzyme structure and function, leading to changes in cell function such as glycolysis, gluconeogenesis, mitosis, and DNA synthesis, among others12,13. Hence it is important to understand the concurrence of elements governing pH maintenance within physiologic boundaries, such as HCO3−, H+, phosphates, albumin, Na+, K+, Cl− lactate, urates, keto acids and others, enabling the preservation of complex and efficient cell functions related to acid–base balance12–14,17.
The Stewart approachIn the late 1970s and early 1980s, Canadian physiologist Peter Stewart proposed a different approach to acid–base equilibrium physiology and disturbances based on a mathematical model in which body fluids were considered as a physicochemical system, supported by three basic principles: (1) Maintenance of the electric charge; (2) maintenance of mass; (3) the law of mass action. The approach derived from the Henderson–Hasselbalch equation does not consider all the factors influencing [H+] and, consequently, does not explain the complex metabolic abnormalities of acid–base balance13,14,18,19. Therefore, in the Stewart approach, HCO3− is presented as a dependent variable, implying that changes in [H+] and, consequently in pH, could only occur through the modification of three variables: (1) PaCO2; (2) total concentration of weak, non-volatile gases ([ATOT]); (3) differences among strong ions (SID)1,2,7,13,14,18,19.
PaCO2 is defined as the pressure exerted by CO2 on arterial blood in the gas mix (independently of each gas), with the physiological implications already mentioned. [ATOT] refers to albumin, inorganic phosphates and HCO3− (weak ions), which are partially dissociated in the blood buffer1,2,7,13,14,18,19. SID is the difference between the sum of plasma concentrations of strong positive charges (cations) and strong negative charges (anions), the most important being sodium, potassium, calcium, magnesium, chlorine and lactate, which are totally dissociated and do not participate in proton transfer reactions. In plasma there is normally cation excess, explaining why SID is a positive value usually ranging between 40 and 48mEq/L (charges and ions present). An increased SID reflects a rise in pH (alkalosis) and a lower SID reflects a drop in pH (acidosis)2,14,20.
In order to determine the metabolic component in acid–base equilibrium using the Stewart model, the strong ion gap (SIG) calculation must be taken into consideration and, for this, the strong ion difference apparent (SIDa) and the strong ion difference effective (SIDe) must be calculated first. SIDa is determined by the formula SIDa=([Na+]+[K+]+[Mg2O+]+[Ca2+])−([Cl−]+[Lactate−]), where the contribution of weak acids is not considered, whereas SIDe is determined by the formula SIDe=[(2.46×10−8)×(PCO2/10−pH)]+[[Albumin]g/L×0.123×(pH −0.631)]+[[Phosphates]mmol/L×0.309×(pH −0.469)], in which weak acids are also included. Therefore, to calculate SIG, the difference between SIDa and SIDe is determined (SIG=SIDa−SIDe) and indicates the presence of other non-measured weak anions such as keto acids, sulphates, urates, citrate, pyruvate, acetate and gluconate2,3,13,15,21–26.
SIG equals zero when only plasma buffers (bicarbonate, albumin and phosphates) are added to [Cl−] and to [lactate−]. In other words, SIG=0, when SIDa=[HCO3−]+[Albumin−]+[HPO32−]2,3,13,21–25.
The Siggaard–Andersen approachIn 1960 Siggaard and Andersen implemented a method using capillary blood to determine acid–base balance based on the Van Slyke equation. This method emphasizes the use of base excess (BE) or deficit, representing the number of additional acid or base milliequivalents needed to be added to a litre of blood in order to normalise pH at 37°C. In this calculation, PaCO2 and pH (measured) are considered. This variable is divided into BE and standard BE (SBE), the difference being that the gas machine calculates the latter based on an estimated haemoglobin concentration of 5g/dl in extracellular fluid6,24,27–33.
There is a direct relationship between SBE and changes in SID and weak acid concentrations. This means that a base deficit (negative SBE) corresponds to a positive SID and reflects the presence of non-measured anions (e.g. lactate), while positive SBE corresponds to a negative SID. Consequently, it represents a reliable measurement of the metabolic component and constitutes a practical tool for clinical application6,24,27–33.
ConsiderationsDerksen et al19. showed that the Henderson–Hasselbalch method may be considered a simplified version of Stewart's general model and that the two are not different despite the fact that the latter considers more variables, because, in the end, the clinical interpretation is the same. When Stewart's theoretical model is compared with the traditional rationale, we find that it offers a clearer understanding of the underlying pathophysiological mechanism, but is no different when it comes to the diagnosis of acid–base imbalances, or the initial therapeutic management19,34–38.
There is no consensus so far regarding prognostic implications, because a higher SID has been associated with unfavourable results in some groups19,39, and base and lactate deficits have been found to be independent mortality risk factors in trauma patients and patients undergoing cardiovascular surgery39,40. On the other hand, Kurtz et al2. concluded that both approaches are quantitatively interchangeable and do not offer diagnostic or prognostic advantages2,20.
Clinical application: step-by-step diagnosis of acid–base imbalancesAcid–base disturbances may be primary, although they are more commonly secondary to diseases such as Diabetes Mellitus, renal failure, seizures, sepsis, gastroenteritis, pancreatic leaks, bowel obstruction, or the use of medications such as isoniazid, furosemide or linezolid. Likewise, other systems may be affected during the course of a disease with acid–base imbalances, including the immune system, and bone resorption and formation abnormalities. However, the pathophysiological mechanisms have not been clearly elucidated5,41,42.
The approach to the diagnosis of acid–base imbalances requires establishing the relationship between the clinical history (vomiting, diarrhoea, oedema, dyspnoea, trauma, transfusions or medications), the physical examination (signs of dehydration or oedema, polypnea, tetany, coma) and blood gas parameters. This requires determining which is the predominant component (respiratory or metabolic), and analysing the consistency of the compensatory mechanism, always bearing in mind that there are physiological overcompensations that lead to mixed or combined acid–base disturbances5,26,43–46.
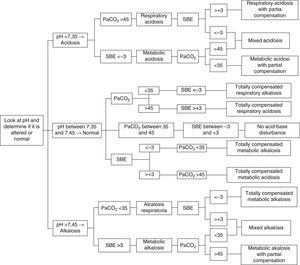
A simple, expedite approach without complex and multiple variables that can estimate metabolic components is of the utmost importance to help with the interpretation and diagnosis of acid–base imbalances. Therefore, we recommend the use of the approach that implements SBE and to follow the algorithm proposed in Fig. 1, with the following analytical sequence:
- 1.
Patient: knowledge and analysis of the patient's clinical context.
- 2.
Primary disorder: determine whether blood pH indicates acidosis (<7.35), alkalosis (>7.45) or if it is within the reference rage (7.35–7.45), where the possibilities are total compensation, or absence of acid–base disturbances.
- 3.
Origin of primary disorder: in accordance with the clinical context, determine whether to analyse the respiratory (PaCO2) or the metabolic (SBE) component in order to determine the origin or the predominance of the acid–base imbalance:
- •
PaCO2 lower than 35mmHg indicates respiratory alkalosis, and PaCO2 greater than 45mmHg indicates respiratory acidosis (reference range between 35 and 45mmHg).
- •
SBE less than −3mmol/L indicates metabolic acidosis, and SBE greater than a +3mmol/L indicates metabolic alkalosis (reference range between −3 and +3mmol/L).
- •
- 4.
Consistency of the compensatory mechanism or mixed disorder (combined): once the origin of the primary disorder is determined (respiratory or metabolic), a determination is made of whether the other component tries to compensate, or totally compensates, the pH (e.g. respiratory acidosis with metabolic alkalosis); or if, on the contrary, it helps perpetuate the disorder (e.g. metabolic acidosis with respiratory acidosis)5,6,26,29,32,43–45,47–50. (Fig. 1).
Step-by-step diagnosis of acid–base disturbances5,6,26,29,32,43–45,47–50.
The following cases will be analysed applying the diagnostic algorithm for acid–base disturbances proposed in Fig. 1:
Case 1: A 35-year-old male patient with a history of alcohol abuse was found unconscious in his apartment by a neighbour. He is taken to the emergency service where he arrives with a blood pressure of 100/60mmHg, heart rate of 124×min, no fever, mild response to painful stimuli. Blood gases are taken on admission, with the following results: pH 6.92, PaCO2 80mmHg and SBE −14.2mmol/L.
According to the proposed algorithm, the first thing to do is to use pH to determine whether there is acidosis, alkalosis or normal balance. In this case, pH is 7.35, corresponding to acidosis. Next is the metabolic component, and BE is less than −3mmol/L, indicating metabolic acidosis. Finally, PaCO2 is greater than 45mmHg, leading unexpectedly to the initial diagnosis of respiratory acidosis, meaning that there is no respiratory compensation. The conclusion from the analysis is a diagnosis of mixed acidosis51.
Case 2: A 72-year-old female patient is admitted to the emergency service with symptoms of fever, lower abdominal pain, dyspnoea, and anuria lasting for 2 days. She has a history of diabetes mellitus type II, treated with metformin. Findings on physical examination include dehydration, tachypnea, heart rate 120×min, blood pressure 110/80mmHg, and no other abnormal findings. The results of blood gases taken on admission are pH 6.82, PaCO2 20.2mmHg, SBE – 30.2mmol/L.
In this case, pH is lower than 7.35, indicating acidosis. BE is less than −3mmol/L, so there is metabolic acidosis. Finally, PaCO2 is found to be less than 35mmHg, leading to the diagnosis of respiratory alkalosis. Based on all the results, we may conclude that the diagnosis is metabolic acidosis with partial compensation (the physiological mechanisms cannot bring the pH within the reference range)19.
Case 3: A 51-year-old female patient admitted to hospital with symptoms of fever, dyspnoea and cough. She had been discharged five days before after presenting with CMV gastroenteritis. She has a history of hypertensive renal failure with renal transplant 15 months before and was given prednisolone, valganciclovir, metoprolol, acetaminophen, mycophenolate mofetil and ranitidine. On physical examination, temperature is 41°C, heart rate 90×min, blood pressure 170/80mmHg, respiratory rate 33×min, and there is left basal crepitation. Blood gases show the following results: pH 7.42, PaCO2 19.5mmHg, BE −10.4mmol/L.
In this case, pH is within the reference value7,42, but given the history of grastroenteritis and renal transplant due to renal failure, the first step is to look for the metabolic component, and the SBE is found at −10.4mmol/L, revealing metabolic acidosis. Then, expecting a compensatory response, a low PaCO2 (19.5mmHg) indicates respiratory alkalosis. Results lead to a final diagnosis of totally compensated metabolic acidosis. It is of the utmost importance to establish an adequate clinical correlation when evaluating blood gas parameters in order to avoid making a wrong diagnosis. In this case, failure to make the correlation would lead to a diagnosis of totally compensated respiratory alkalosis19,52.
ConclusionsAlmost all the physiological variables available in plasma are used for analysing the acid–base equilibrium with the Stewart model, although the model is difficult to apply in clinical practice. The traditional Henderson–Hasselbalch approach may be considered a simplified and readily measured model, which does not change the diagnosis or the initial management.
BE is calculated by the blood gas machine from values like pH and PaCO2, the same used in the traditional approach, and it provides an accurate estimate of the metabolic component of the acid–base equilibrium calculated by the Stewart method. So, for practical purposes, the three variables – pH, PaCO2, and SBE – may be used to achieve a correct diagnosis of acid–base disturbances, using the proposed algorithm (Fig. 1) in clinical practice.
Conflicts of interestThe authors have no conflicts of interest to declare.
FundingThe authors did not receive sponsorship to undertake this article.
Please cite this article as: Aristizábal-Salazar RE, Calvo-Torres LF, Valencia-Arango LA, Montoya-Cañon M, Barbosa-Gantiva O, Hincapié-Baena V. Equilibrio Ácido-Base: el mejor enfoque clínico. Rev Colomb Anestesiol. 2015;43:219–224.