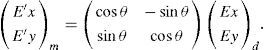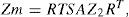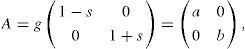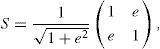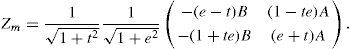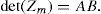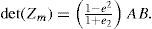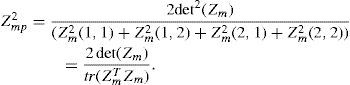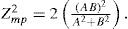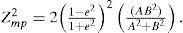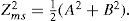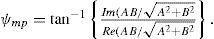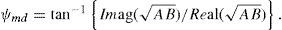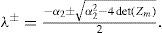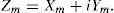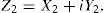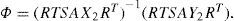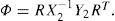Las impedancias serie y paralelo del tensor magnetotelúrico se evalúan en relación con su relativa inmunidad a las distorsiones galvano-eléctricas. Las respuestas distorsionadas se modelan utilizando la descomposición del tensor en términos de giro, cizalla estática y rumbo de Groom y Bailey. Estos cuatro parámetros, junto con las impedancias sin distorsión, normalmente se consideran incógnitas y se obtienen de los datos mediante la solución de un problema inverso. En el presente trabajo utilizamos la descomposición como un modelo directo para simular sondeos distorsionados. Partiendo de respuestas 2-D sin distorsiones, el tensor se distorsiona suponiendo valores arbitrarios de giro, cizalla, estática y rumbo. Por definición las impedancias serie y paralelo son inmunes al rumbo porque son invariantes ante rotación. Adicionalmente, la impedancia serie es inmune a giros y a cizalla, y la impedancia paralelo sólo a giros. La impedancia paralelo depende de cizalla en la forma de un factor que desplaza hacia abajo las curvas de amplitud. Por otro lado, el efecto de la estática en ambas impedancias es más complicado que en el tensor mismo porque no se puede corregir con un simple desplazamiento de las curvas. En términos generales, hay un balance positivo por parte de las impedancias serie y paralelo sobre las respuestas te y tm porque los invariantes filtran varias distorsiones. Se muestra que la condición de invariante no es suficiente para tener inmunidad a cualquiera de las distorsiones. Se utiliza para esto los valores característicos de Eggers, los cuales son inmunes sólo al rumbo, como todos los invariantes. Se muestra además que la invariancia tampoco es una condición necesaria para ser inmune a las distorsiones, según lo atestigua el tensor de impedancia, el cual depende del rumbo pero está libre de las demás distorsiones. Los desarrollos se ilustran utilizando sondeos de los conjuntos de datos coprod2s1, coprod2 y bc87.
The series and parallel impedances of the magnetotelluric tensor are appraised in relation to their relative immunity to galvanic electric distortions. The distorted responses are modeled using the Groom-Bailey decomposition of the tensor in terms of twist, shear, statics and strike direction. These four parameters and the undistorted responses are normally considered as unknowns, and are obtained from field data through the solution of an inverse problem. In the present work we use the decomposition as a forward model to simulate distorted sounding curves. Starting with undistorted 2-d te and tm responses, the tensor is distorted by assuming arbitrary values of twist, shear, static and strike direction. By default, both series and parallel responses are immune to the strike direction because they are invariants under rotation. In addition, series responses are immune to twist and shear and parallel responses only to twist. The dependence of the latter on shear is in the form of a real factor that shifts downwards the amplitude curves. On the other hand, the effect of statics on both series and parallel responses is more complicated than that on the impedance tensor because it cannot be accounted for by a simple shift of the curves. On the whole, there is a positive balance on the part of the series and parallel impedances over the te and tm responses because some of the distortions are filtered out by the invariants. It is shown that invariance is not sufficient to be immune to any of the distortions. The example chosen is Eggers’ eigenvalues, which are immune only to the by-the-fault strike direction. Invariance is not necessary either, as evidenced by the phase tensor, whose elements depend on strike but are immune to all distortions. The derivations are illustrated using soundings from the synthetic coprod2s1 and field-recorded coprod2 and bc87 data sets.
The series impedance is defined in terms of the sum of squares of the elements of the impedance tensor, and the parallel impedance in terms of the sum of squares of the elements of the admittance tensor, the inverse of impedance. Both quantities, series and parallel, are scalar measures of impedance and unlike the individual elements of the tensor, are invariant under rotation of the system of coordinates. Other properties include that series is particularly sensitive to underground resistors and that parallel is particularly sensitive to underground conductors (Romo et al, 2005). Beside these properties very little is known about these invariants. Two-dimensional (2d) inversions of synthetic and field data throw very similar models to those obtained using the traditional te and tm sounding curves. In particular, we have noticed that using te and tm data that are corrected for galvanic effects produces results suspiciously similar to those obtained using uncorrected series and parallel responses (Antonio-Carpio et al., 2011). This could be due to a mere accident or it could be an indication of unknown properties of the two invariants. To clarify this issue it is necessary to make a rigorous appraisal of the effect of galvanic distortions on the series and parallel invariants.
The impedance tensor of a regional 2-d conductivity structure can be severely distorted by local 3-d structures that are inductively small, where inductively small means that their size is smaller than the skin depth. The distortions are due to perturbations of the regional electric field by local 3-d charge distributions, and to perturbations of the regional magnetic field by also local current distributions. In principle, the local 3-d structures can be included in the interpretation process as part of the sought model of the Earth. However, this is seldom done. Rather, the process is divided into two steps: the distortion issue is first settled in some way and then the undistorted data is properly interpreted. It is possible to deal with distortions separately because they are not completely arbitrary but follow some rules. The most important fact is that the impedance tensor distorted by electric effects can be simulated by a real tensor multiplied by the undistorted impedance (Berdichevsky and Dmitriev, 1976a; Bahr, 1988). This means that the basic physics and its mathematical representation are well understood. However, what matters in practice is to recover the undistorted impedances. To this end, Groom and Bailey (1989) proposed a factorization of the real distorting tensor that they call a physical decomposition. They introduced two concepts called twist and shear, which together with strike direction and static factors completely simulate distorted experimental impedances. Twist and shear are physically meaningful effects on electric fields that distort the impedance in distinctly mathematical terms. In fact, they can be distinctly identified in the numerical solution of the nonlinear inverse problem proposed by Groom and Bailey (1989). While it is true that the factorization is not unique, as noted by Caldwell et al. (2004), many practical applications attest for the validity of the model to simulate distorted impedances (e.g. Ledo and Jones, 2001).
When computing series and parallel impedances we do not know how the distortions propagate from the elements of the tensor to the invariants. Using Groom-Bailey’s factorization the procedure to find out is straightforward but the result is far from trivial. The paper is written in a tutorial-review manner including results for the determinant, the classical invariant, and for Egger’s (1983) invariant eigenvalues. This last application illustrates that the result for series and parallel modes is not due solely to their invariant character. A final discussion about the phase tensor of Caldwell et al. (2004) gives perspective to the other results, illustrating that invariance is not a necessary condition to avoid distortions.
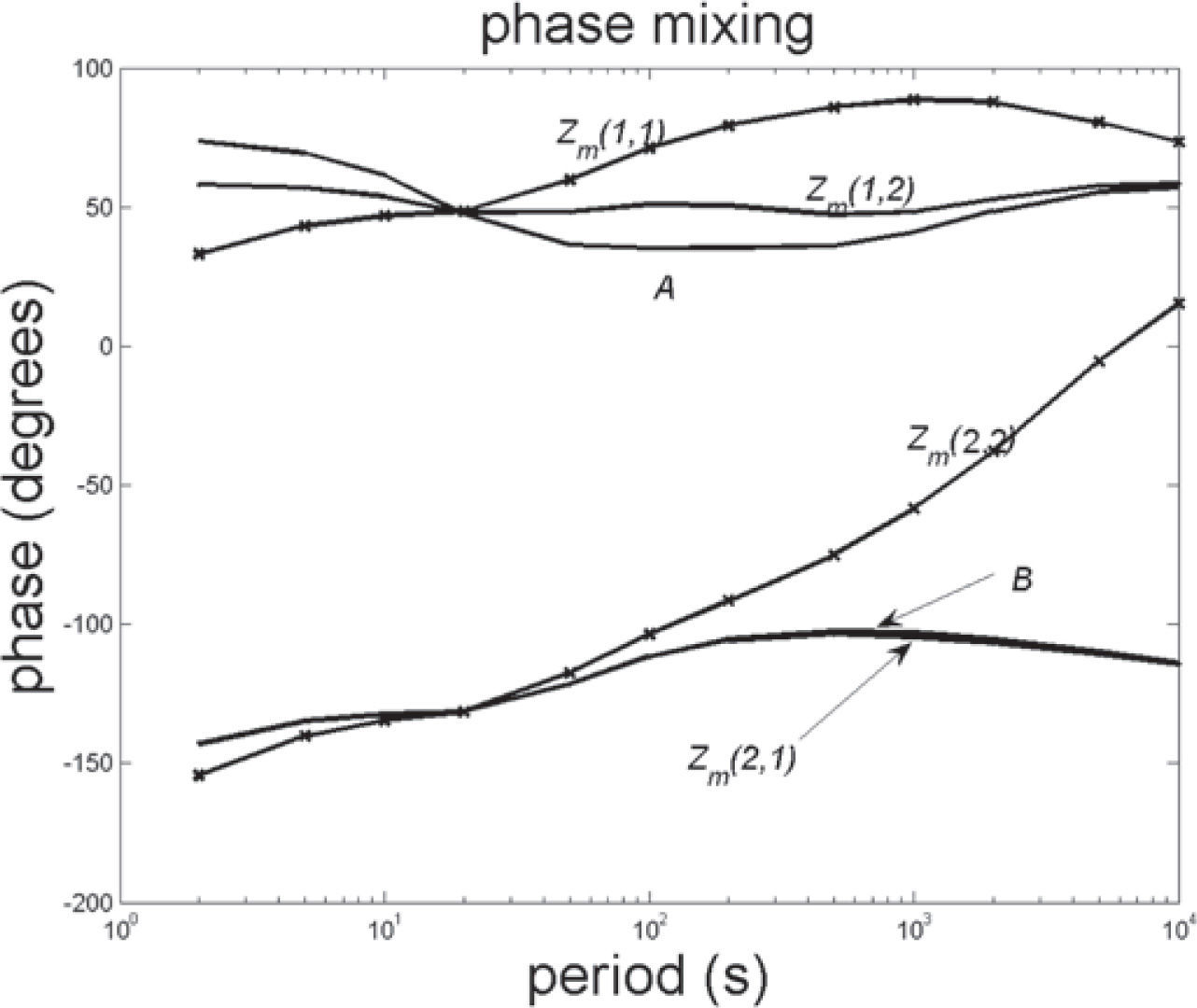
MethodologyThe algebra of distortionsAssume a two-dimensional (2-d) electrical resistivity distribution subject to plane electromagnetic waves from above. Placing the x-axis of a Cartesian coordinate system along strike, the components of the electric and magnetic fields are related as
The components Ex and Hy correspond to the TE or E-polarization mode and A is the respective impedance. Accordingly, Ey and Hx defines the TM or H-polarization mode with its impedance B. It has been established that the distortions of the electric field can be modeled using a real tensor C as
Behind the simplicity of this equation there are extensive studies that led to its development (e.g. Berdichevsky and Dimitriv, 1976; Bahr, 1988). Substituting (1) in (2) we obtain
If we now rotate the system of coordinates by an angle θ the components of the distorted electric field in the rotated system are
The components of the magnetic field need to be rotated too. Solving for the unprimed coordinates and substituting the result along with equation (4) back into equation (3), the resulting equation relates the electric and magnetic fields in the primed coordinates. The corresponding impedance is
Notice that in equation (5) the columns of the distorted impedance before rotation have the same phase because the distorting factors are real. After rotation the elements of Zm are all different combinations of A and B and their phases are mixtures of the phases of the two modes. This is illustrated in Figure 1 using the impedances of sounding # 11 of the synthetic data set coprod2s1 made available in MTnet by Varentsov (1998). The calculations were made using c1 = 1.97, c2 = −0.77, c3 = 0.35 and c3 = 0.64.
A and B correspond to the te and tm modes of sounding # 11 of the synthetic data set coprod2s1 made available in mtnet.dias.ie by Varentsov (1998). When θ ≠ 0 in equation (5) the elements of the resulting impedance all are mixtures of the phases of A and B. Calculations were made using c1= 1.97, c2 = −−0.77, c3 = −0.35 and c4 = 0.64. The graphs correspond to the phase of the impedance.
Groom and Bailey (1989) proposed to factorize or decompose the tensor C such that
where
and
Notation is changed a little as indicated in equation (6a). The tensor A accounts for anisotropy and the scalar g for the site gain. All together they simulate static shifts on impedances A and B. We simply use a and b as the corresponding factors, instead of the more elegant arrangement with g and s. Tensor T twists the electric field so the parameter t is called twist. Tensor S produces a shear-like effect so the parameter e is called shear. R is simply a rotation matrix to account for the coordinate system to be off an angle θ from strike. Twist and shear are expressed in degrees such that t and e are the tangent of the corresponding angles.
Figure 2 illustrates what each of the operators does to an undistorted unitary electric field. The undistorted field varies from (0,1) to (0, −1) to cover different initial directions to allow a pattern to emerge. Each operator can be thought to be the distortion tensor in equation (2) as applied to an undistorted electric field. Twist is a simple rotation of the electric field similar to the rotation of coordinates, and it could be thought to be an overlap of the latter. However, this is not so because the rotation of coordinates involves both electric and magnetic fields or, equivalently, the whole impedance tensor and not only the electric field. Another important comment about this factorization or decomposition is that it does not reduce or increase the number of distorting parameters; it just spreads them in a useful way for their recovery from the elements of Zm.
The effect of the individual distortion tensor T, S and A are illustrated in a), b) and c), respectively. The figures illustrate what each of the operators does to an undistorted unitary electric field shown in a). The undistorted field varies from (0,1) to (0, −1) to cover different initial directions to allow a pattern to emerge. Each operator can be thought to be the distortion tensor in equation (2) as applied to a unitary and undistorted electric field. The figure is inspired in a similar one in Groom and Bailey (1989).
The impedance tensor, distorted or undistorted, can be mathematically transformed into an exactly equivalent set of 8 real numbers. We consider in the next sections some of these transformations. Groom and Bailey (1989) factorization, although mathematically expressed, is not a mathematical decomposition in the same sense that these others are. It is more a convenient proposal to allow for the distortions to be estimated from the elements of Zm through an inverse procedure. They do it by neutralizing the two distortion factors a and b due to static, simply by placing A next to Z2 The unknowns now become aA and bB.
This reduction in the number of unknowns accomplishes two things. Zm on the left side of equation (6) provides with 8 real numbers as data, and on the right side we have 9 unknowns: θ, t, e, a, b and four real numbers to make Z2. This means that without this absorption the inversion cannot even be attempted. However, this is not the most important reason because the relative number of data can be increased by using many frequencies and by forcing θ, t and e to be the same for all frequencies. The second reason is more fundamental because it cannot be dealt with except by doing what they did. It is universally acknowledged that static distortions factors cannot be determined from the impedance tensor alone, and that independent information is needed to resolve them (e.g. Pellerin and Hohmann, 1990; Ledo et al., 2002). By absorbing the static factors into A and B the problem is solvable. We can mimic the recovery of static shifted curves by explicitly solving for them in equation (6). The recovery equation is
First we compute a distorted Zm using equation (6). The undistorted Z2 is the same sounding used in Figure 1. The distortions are as follow: two different static factors a=2 and b=3 in one case and a=3 and b=2 in the other, and the same θ, t and e for both cases. The distorted, undistorted and recovered curves are shown in Figure 3. Notice that the recovered curves are scaled versions of each other, but the disturbed ones are not. Note also that the recovered curves are scaled versions of the original TE or A curve.
The recovery of undistorted TE impedances using equation (7) is correct save for the static factors. The distorted Zm is computed using equation (6) and the indicated distorting parameters of twist, shear and strike. The undistorted Z2 is the same sounding used in Figure 1. Notice that the recovered curves are scaled versions of each other, but the disturbed ones are not. Note also that the recovered curves are scaled versions of the original TE or A curve. In this instance it is pretended that we know the distorting parameters of twist, shear and strike in equation (7).
In general, Zm depends on θ, t, e, a and b, as indicated by equation (6). In other words, all of them are needed to ft experimental impedances. The Groom and Bailey (1989) approach does not use the static factors to fit Zm, but this does not mean that Zm is immune to them. In other words, the unprocessed Zm is immune to none of the distorting parameters: twist, shear, static and strike. This is a natural starting point to analyze other responses derived from Zm that are immune to one or more types of distortions.
When dealing with invariants, we can dispense of the rotation matrix and its transpose and use the simplified expression
This equation follows from (6) by carrying out the multiplications. Again, we assume that a is absorbed in A and b in B.
ResultsImmunity to twistThe first invariant to examine is the classical determinant of the impedance tensor (Berdichevsky and Dimitriv, 1976). In the absence of distortions, the determinant is simply given as
In general, when the four elements are present in the tensor, the determinant is given as
To determine the effect of distortions we must substitute equation (8) into equation (10).
The result is
The twist parameter t cancels in the algebra but not the shear parameter e. The effect on the determinant is equivalent to modify the static factors on either A or B, or both. With no static effects the amplitude of the determinant would still be smaller than the actual one if shear distortions are present. On average, we might expect the static factors of the determinant to be biased towards values less than unity.
An invariant closely related to the determinant is the parallel impedance (Romo et al. 2005) which is given as
Making t=e=0 in equation (8) and using equation (12), the undistorted parallel impedance is
On the other hand, the general case reduces to
Again, the distorted impedance is immune to twist, but not to shear and, of course neither to static, which is hidden in A and B. The effect of shear is most effective on the parallel impedance than on the determinant because of the square. This is illustrated in Figure 4 for both invariants. The quantity plotted is the traditional apparent resistivity |Z|2/(ωµ0), where ω stands for angular frequency and µ0 is the permeability of free space.
Immunity to twist and shearIt is interesting how in the previous section twist separates quite neatly from shear, to leave only the latter as an active distorter. In this section we report an invariant that leaves no trace of both twist and shear. This invariant we call the series equivalent (Romo et al., 2005) corresponds to half the sum of squares of the elements of the impedance tensor (Szarka and Menvielle, 1997). This is
When t=e=0, this reduces to
In general, substituting equation (8) in (15) leads to
It turns out that this reduces to the undistorted series impedance, which means that this type of invariant response is immune not only to strike, but also to both twist and shear. Of course, it is still affected by static because A and B have absorbed a and b, the two static factors.
The distorted parallel impedance given by equation (14) differs from the undistorted impedance by a real factor that depends on shear. This implies that the corresponding phase is immune to shear, and because the complex impedance was already immune to strike and twist, this implies that the phase of the parallel impedance is immune to all three distorting parameters. The phase is given as
Immunity to strike, twist and shear isolates static effects. It can be appreciated in both equation (16) and (18) that static factors will produce phase mixing. This is illustrated in Figure 5 for the phase of the series impedance. Static effects, absent in the phase of the TE and TM modes, can severely distort the phase of the series impedance. Care must be taken before or while inverting the data. The same applies to the phase of the parallel impedance, for while the factors cancel in the product AB, they do not within the square root sign.
Immunity to strike, twist and shear isolates static effects as in the case of equation (16) for the series impedance. Unfortunately, if A and B are affected by statics (a and b different from unity) this produces a static-dependent phase mixing that is not present in the original impedances A and B. This is illustrated in this figure. Static effects, absent in the phase of the TE and TM modes, can severely distort the phase of the series impedance. The same applies to the phase of the parallel impedance, for while the factors cancel in the product AB, they do not within the square root sign in equation (18).
Equation (11) indicates explicitly that the determinant is affected by shear through a multiplicative factor. On the other hand, implicitly it is affected by static though another factor (ab), which comes from the absorption of a and b into A and B. All together they compose a single factor that vanishes when determining the phase of the determinant. That is
The phase of the determinant is then immune to shear and static, along with twist and strike that shares with the complex determinant. We have arrived at a standstill, for there is nothing that we can vary that alters the phase of the determinant.
Invariance is not sufficientThe twist tensor as defined in equation 6(b) is a rotation matrix that changes the direction of the electric field. This may seem to indicate that all invariants are immune to twist, as are the three invariants analyzed so far. However, this is not the case. Consider the two invariant eigenvalues of Eggers (1983), which are given by the quadratic formula
where
To see how the distortions affect these invariants we need to use the general expression for Z given in equation (10). Let us first substitute Z in (20) when t=e=0. The result is
In this case the eigenvalues reduce to either A or B and in the distorted case to
This is about the minimum expression one can obtain for the distorted eigenvalues. It can be observed that t and e are present in the expression and that there is no possible way that those in the numerator cancel out with those in the denominator. It is also clear that the effect of twist and shear cannot be absorbed as equivalent static factors on A and B, although they can in several places in the expression. The factor (1+te) multiplies B and (1-te) multiplies A, both outside and within the square root. However, the absorption cannot be completed for the product AB within the square root. Summarizing, the two eigenvalues are not immune to any of the distortions. Figure 6 illustrates this with the phase of the two eigenvalues using different factors for twist, shear and static. Notice that the curves show phase mixing of the original phases of A and B.
Eggers’ eigenvalues, the invariants defined by equation (23) are not immune to any of the distortion parameters of twist, shear and statics. This is illustrated here with the phase of the two eigenvalues. This case demonstrates that invariance under rotation is not a sufficient condition for immunity to any of the other distortions.
We now come to a very interesting response that can be derived from the impedance tensor. It is called the phase tensor and was proposed by Cadwell et al. (2004). This response is immune to twist, shear and static and it is not invariant. The measured impedance is separated into its real and imaginary parts as
If Zm were a simple complex number, the tangent of its phase would be its imaginary part divided by its real part. In other words, the tangent of its phase would be the inverse of its real part multiplied by its imaginary part. But Zm is not a simple complex number, it is a tensor. However, nothing prevents to multiply the inverse of the real tensor by the imaginary tensor. That is
The product is a dimensionless tensor whose elements can be interpreted as the tangent of an angle. So far there is nothing impressive about this. However, let us now enquire about the effect of the different distortions on this dimensionless tensor. The undistorted tensor can be written as
Substituting this into (6) and doing the operation as in (25) we have that
Performing the operations there results that T, S and A cancel with their corresponding inverses. The expression reduces to
The phase tensor depends only on strike. This is a very important property because it allows obtaining the strike direction independently of the distorting parameters. Figure 7 illustrates the dependence of diagonal elements of the phase tensor as strike is varied.
Contrary to the invariants, the phase tensor depends on strike and at the same time it is immune to all other distortions. This is a very important property because it allows obtaining the strike direction independently of the distorting parameters. This figure illustrates the dependence of the diagonal elements of the phase tensor as strike is varied. The phase tensor is an example of a response that is not invariant but is immune to distortions. This result, together with that of Figure 6, imply that invariance is neither sufficient not necessary for immunity to distortions, and that each response must be evaluated on its own.
The properties of the determinant in relation to distortions are well known (e.g. Arango et al., 2009) and derive from the fact that if Zm = CZ2, then det (Zm) = det (C) det (Z2). Given that C is real the phase of det (Zm) is the same as the phase of det (Z2). Stated in this way this result is more general than that of equation (11), which assumes that the undistorted impedance is 2-D. The results for the series and parallel impedances derived above also assume a 2-D undistorted impedance. This means that for real data, seldom fully 2-D, we should expect the results to hold exactly for the determinant and only approximately for series and parallel. This issue is explored in the next three figures. First, we present a reference case using synthetic 2-D data. What we have done is to distort the original TE and TM data using equation (6) and then compute the three invariants of Zm. It can be observed in Figure 8c and 8d that both apparent resistivity and phase curves of the series impedance are immune to the distortions. The comparison is made for three shears, 0, 30 and 40 degrees. Strike direction and twist are not recorded because these and the other results presented below are exactly the same regardless of the value of these parameters. According to theory the only distortion left is due to shear and this is what Figure 8 shows. The determinant and the parallel apparent resistivities, as shown in Figure 8a and 8c, suffer a downward shift as predicted by equations (11) and (14), respectively. The shift being more pronounced the larger the shear, and the shift of the parallel resistivity being larger than that of the determinant, as it should be according to theory. It can also be observed in Figures 8b and 8c that the corresponding phases are not affected by shear.
Synthetic te and tm data are distorted using equation (6) and then invariants of Zm are computed. It can be observed in b) and d) that the phases of the determinant (ψd), series (ψs), and parallel (ψp) impedances are immune to distortions, in agreement to theory. The corresponding amplitudes shown in a) and c), also in agreement to theory, are scaled versions of the originals (shear = 0 degrees). This includes the series impedance which is immune to this scaling due to shear. Strike direction and twist are not recorded because the results are exactly the same regardless of the values of these parameters.
The results using field data are presented in Figures 9 and 10. We chose soundings from the coprod2 and bc87 data sets (Jones, 1993a, 1993b). It can be observed that in both cases the corresponding curves behave very much like those using synthetic data. We used equation (6) to further distort both soundings with different strike directions, twists and shears and then computed the three invariants. It can be observed that the phases of the three invariants are immune to the changes of the distorting parameters, implying that they are free of distortions except for the implicit static factors. Notice that the phases of the determinant are exactly the same for the three shears (Figures 9b and 10c) and that those of the series and parallel depart a little from each other (Figures 9d and 10d). As mentioned before, this is because the determinant makes no assumption about dimensionality while the other two assume a 2-d undistorted impedance. Overall, the phases for the three shears are reasonably close considering that a shear of 40 degrees is close to the maximum of 45 degrees.
Results for the determinant, series and parallel impedances using field data from the COPROD2 data set, show that the corresponding curves behave very much like those of Figure 8 using synthetic data. We used equation (6) to further distort both soundings with different strike directions, twists and shears and then computed the three invariants. It can be observed that the phases of the three invariants shown in b) and d) are immune to the changes of the distorting parameters, implying that they are free of distortions except for the implicit static factors. Notice that the phases of the determinant are exactly the same for the three shears and that those of the series and parallel depart a little from each other. As mentioned in the main text, this is because the determinant makes no assumption about dimensionality while series and parallel assume a 2-D undistorted impedance. The amplitudes shown in a) and c) are scaled versions of the originals (shear = 0 degrees) in agreement to theory. This includes the series invariant which is immune to this scaling due to shear. Overall, the phases for the three shears are reasonably close considering that a shear of 40 degrees is close to the maximum of 45 degrees.
Results for the determinant, series and parallel impedances using field data from the BC87 data set, show that the corresponding curves behave very much like those of Figures 8 and 9. It can be observed in c) that there is already a significant downwards shift of the parallel amplitude with respect to series that is not related to the added shear scaling. As discussed in the main text, this could be due to a preexisting shear distortion or to static effects. The rest of the curves in a), b) and d) follow the same patterns as in Figures 8 and 9. Notice that, as in the other tests, the series amplitude is immune to the scaling introduced by the shear distortion as predicted by equation (17).
The example drawn from the bc87 data set illustrates an important point about what is removed and what remains to be done to completely clean the data. Notice that at short periods the series and parallel apparent resistivities (Figure 10c) are both horizontal for the null shear, and that the latter is depressed in relation to the former. This suggests that the parallel apparent resistivity curve has an intrinsic shear distortion that is depressing it from the undistorted series resistivity. This would lead us to shift the curve upwards and leave the phase unchanged to undo the effect of shear. However, the fact is that we do not know whether this shift is due to shear or static. The parallel resistivity curve could also be lifted by playing with the implicit static factors, in which case the phase curve would have to be modified according to equation (18). Deciding which of the two is the proper way to proceed is outside the scope of this first communication. The present results are encouraging to continue the process of advancing our knowledge of the series and parallel invariants, whose properties are just beginning to be understood.
ConclusionGalvanic distortions of magnetotelluric data can be neutralized by some invariants of the impedance tensor. Except for strike direction, invariants are devised without any thought about distortions. However, as it turns out for the case of 2-D data, the 3-D distorting effects can gradually be neutralized by an also gradual averaging process. Series and parallel impedances are averages of the tensor elements and, in turn, the determinant is an average of the series and parallel impedances. This gradual immunity is summarized in Table 1. The new results include: a) The amplitude of the series impedance is affected only by statics, being immune to twist, shear and strike, and so are the phases of series and parallel; b) the amplitude of the parallel impedance is immune to twist and strike but not to shear and statics. These results place the series and parallel impedances midway between invariants that are not immune to any of the distortions, like Egger’s eigenvalues, and the phase of the determinant which is immune to all of them.
The table summarizes the immunity of the different responses to the four distorting parameters, strike, twist, shear and statics.
| Strike | Twist | Shear | Statics | |
|---|---|---|---|---|
| Eggers’s eigenvalues | yes | no | no | no |
| Parallel amplitude | yes | yes | no | no |
| Determinant amplitude | yes | yes | no | no |
| Series amplitude | yes | yes | yes | no |
| Series phase | yes | yes | yes | no |
| Parallel phase | yes | yes | yes | no |
| Phase tensor | no | yes | yes | yes |
| Determinant phase | yes | yes | yes | yes |
We would like to thank CONACYT for Grant # 47922. We would also like to thank Alan Jones for providing the COPROD2 and BC87 data sets, and to the anonymous reviewers for useful comments and suggestions.