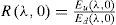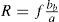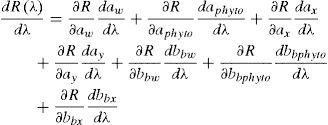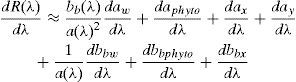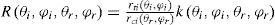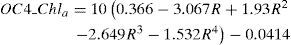Spectral Reflectance Analysis of the Caribbean Sea

Resumen

En este trabajo se analizaron curvas de reflectancia espectral mediante el método de derivada. Los espectros de derivada de la reflectancia revelaron picos ocultos tanto en curvas de reflectancia y absorción del agua del Mar Caribe y de elementos contenidos en ella. Las curvas de reflectancia mostraron un predomínio del color azul (400–500 nm), el cual es característico de aguas oligotróficas del Caribe, los picos de absorción están influenciados por las propiedades ópticas de la clorofila a y el agua de mar. Las curvas de reflectancia mostraron respuesta espectral similar. En este artículo se analizaron los espectros de reflectancia del agua marina en 31 estaciones en el Mar Caribe en el verano de 2001.

Abstract

Reflectance spectral curves were analysed by a derivative method. Derivative reflectance spectra revealed concealed peaks of both reflectance and absorption curves of Caribbean seawater and elements contained within it. Reflectance curves showed a predominant blue colour (400–500 nm) characteristic of Caribbean oligotrophic waters, conspicuous peaks result from the optical properties of chlorophyll a and seawater. Reflectance curves had a similar spectral response. This paper analyses reflectance spectra of surface seawater at 31 stations in the Caribbean Sea during the summer of 2001.

Introduction

Sea surface reflectance R(λ) is operationally defined as the ratio between upwelling irradiance E_u(λ,0) and the downwelling irradiance E_d(λ,0) at wavelength λ at the surface (depth Z = 0) and is expressed as:

The parameter E_u(λ,0) possesses information on the sea water and the dissolved and particulate matter contained within it, whilst E_d(λ, 0) stands for the total incoming irradiance. Changes of ocean colour are determined by the spectral variations of the reflectance R(λ, 0).

The operational expression of Equation (1) can be related to physical properties of water. Many models of radiative transfer are useful for establishing a relationship between R(λ) and the absorption (a) and backscattering (b_b) coefficients (e.g. Morel and Prieur 1977, Gordon and Morel 1983). Both coefficients are influenced by the optical characteristics of pure sea water and those of the particulate and dissolved substances present in variable amounts in the sea. Thus, reflectance spectra are a consequence of absorption and backscattering processes due to water (w), phytoplankton (phyto), carbon dissolved organic matter or CDOM (y) and non-phytoplankton particles (x). Hence, any interpretation of the reflectance must consider the optical properties of these substances.

Morel and Prieur (1977) proposed a simple relationship for the reflectance of oceanic waters:

Where f is a function of solar altitude and the scattering phase function (Gordon et al., 1988). Thus, f can take different values depending on the angle of measurement. Historically, it had the value of 0.33 at the zenith angle of incident light (Morel and Prieur, 1977; Kirk 1984); however, other authors have estimated the f value for different angle intervals (e. g. Hirata and Højerslev, 2008). Nonetheless, this equation is only valid for waters where b_b <_b follow the principle of superposition (Sathyendranath and Morel 1983).

Experimental data and theory indicate that, under most conditions, values of b_b(λ) are relatively small compared to values of a(λ) and decrease monotonically with respect to wavelength (Gordon and Morel 1983).

In order to analyze reflectance spectra a number of techniques have been employed. Thus, ratios and differences have traditionally been used in ocean colour studies, although they are restricted to a small number of spectral bands.

Nowadays, the advent of high-spectral-resolution sensors requires the use of powerful analytical methods of study such as the derivative analysis. The derivative method undertakes many of the problems of quantitative analysis more effectively than ratios and differences by considering a larger amount of data, which stands for more information potentially available (Jia et al., 2008). Derivative analysis has been applied to a different kind of spectra obtained by high spectral-resolution sensors (e. g., Evangelista et al., 2006). These sensors are characterised by having a bandwidth less than 5 nm and/or more than 100 spectral bands. Thus, high-spectral-resolution sensors can provide information about smaller spectral variations than coarse bands do. The derivative method has been used for minimizing low-frequency background noise and for resolving overlapping spectra (Butler and Hopkins 1970). This method has successfully been applied in aquatic remote sensing for studying suspended solids in water (Chen et al., 1992), Goodin et al., 1993); Hunter et al., 2008) and detecting photosynthetic algal pigments (Aguirre-Gómez et al., 2001; Han, 2005), among other topics.

In general, data provided by high-spectral-resolution sensors mounted on an aircraft, on a satellite, and on a field spectroradiometer, can be useful for testing new types of algorithms designed to analyse the reflectance of seawater. Particularly, data provided by field spectroradiometers are important in remote sensing studies because they may simulate those obtained through sensors mounted on aircraft or satellites. Additionally, these measurements are essential for determining the atmospheric effect between the airborne or spaceborne remote sensor and the ground, instead of using models for removing atmospheric influences (Philpot, 1991). Finally, under certain circumstances, it could be better to use ship-based information because of higher confidence of in situ related data.

Consequently, the in situ optical properties, as calculated by equation (2), can be related to ocean colour observations through reflectance information on the marine surface as measured by equation (1).

The aim of this study is to assess the potential of derivative analysis applied to high-spectral-resolution ship-based spectroradiometer data for retrieving information about photosynthetic pigments. Due to the lack of simultaneous in situ measurements of pigments, satellite-derived chlorophyll-a concentration was estimated to validate the spectral reflectance curves.

Derivative reflectance spectra

According to equation (2), the solar radiation reflected from the sea surface depends on absorption and backscattering processes. Both processes are dependent on the water itself, phytoplankton, particulate and dissolved matter (Kirk, 2011). Thus, considering all of these components, the derivative reflectance spectra can be expressed as the rate of change of reflectance with wavelength dR(λ)/dλ. Therefore, by estimating the complete differentiation of R = R(a_w, a_phyto, a_x, a_y, b_bw, b_bphyto, b_bx, λ), we obtain

where the partial derivatives are an implicit function of λ.

Assuming R(λ) ≈ b_b(λ)/a(λ) and by calculating the partial derivative for each component, and substituting in the equation (3), we get:

This equation relates the slope of a directly measured reflectance spectrum at any wavelength to each of the components. Consequently, it is possible to iteratively calculate high-order derivatives. Thus, in principle, the presence of phytoplankton pigments, suspended and organic matter within the sea water can be estimated by considering reflectance spectrum components as mutually independent.

Study area

The Caribbean Sea is the largest sea adjacent to the Atlantic Ocean. It has an upper mixed layer of about 50 m, which quickly responds to atmospheric forcing. Circulation at this layer is controlled by the Caribbean Current, which substantially contributes to heat advection from the tropic to mid-latitudes. The physical characteristics of the Caribbean Sea have been studied from various perspectives (e.g. Gordon, 1967; Etter et al., 1987; Kinder et al., 1985; Müller-Karger et al., 1989). The Caribbean Sea is considered to be an oligotrophic area. This can be supported in terms of its efficiency of light utilisation. An areal efficiency e_A (ratio of areal photosynthetic rate to the total Photosynthetically Active Radiation), in a range of 0.02%-0.07%, was reported for the Caribbean Sea, which can be interpreted as a relatively low amount of phytoplankton within the euphotic zone (Morel, 1978). When the pycnocline is deeper than about 100 m, the deep chlorophyll maximum is extremely weak. However, the literature contains few studies of the bio-optical characteristics of the area, measured with spectral radiometers and apparently no reports of its reflectance properties (e.g. Clark and Ewing, 1974; Farmer et al., 1993).

The reflectance characteristics of oceanic waters have been studies by a number of authors (e.g. Wilson and Kiefer, 1979; Morel et al., 1995). With the advent of high spectral resolution sensors the analysis of reflectance properties has been significantly improved.

The Bi-directional Reflectance Factor (BDRF) method proposed by Milton (1987) has been widely used on lakes and lagoons (e.g. Dekker et al., 1991; Aguirre-Gómez, 2001) and on oceanic sites (e.g. Bidigare et al., 1989; Aguirre et al., 2001). One of the advantages of using high spectral resolution sensors is the potential for applying sophisticated algorithms, such as the derivative method, to nearly continuous curves. Particularly, it is a powerful tool for detecting information concealed in spectral reflectance curves. Thus, the main objective of this study is to analyze the spectral reflectance characteristics of the Caribbean Sea through the derivative method.

Material and Methods

Hydrographical, meteorological and optical measurements were performed over the Caribbean Sea during the oceanographic/meteorological cruise ECAC-3, onboard the R/V Justo Sierra (Universidad Nacional Autónoma de México, UNAM) from July 6 to 26, 2001.

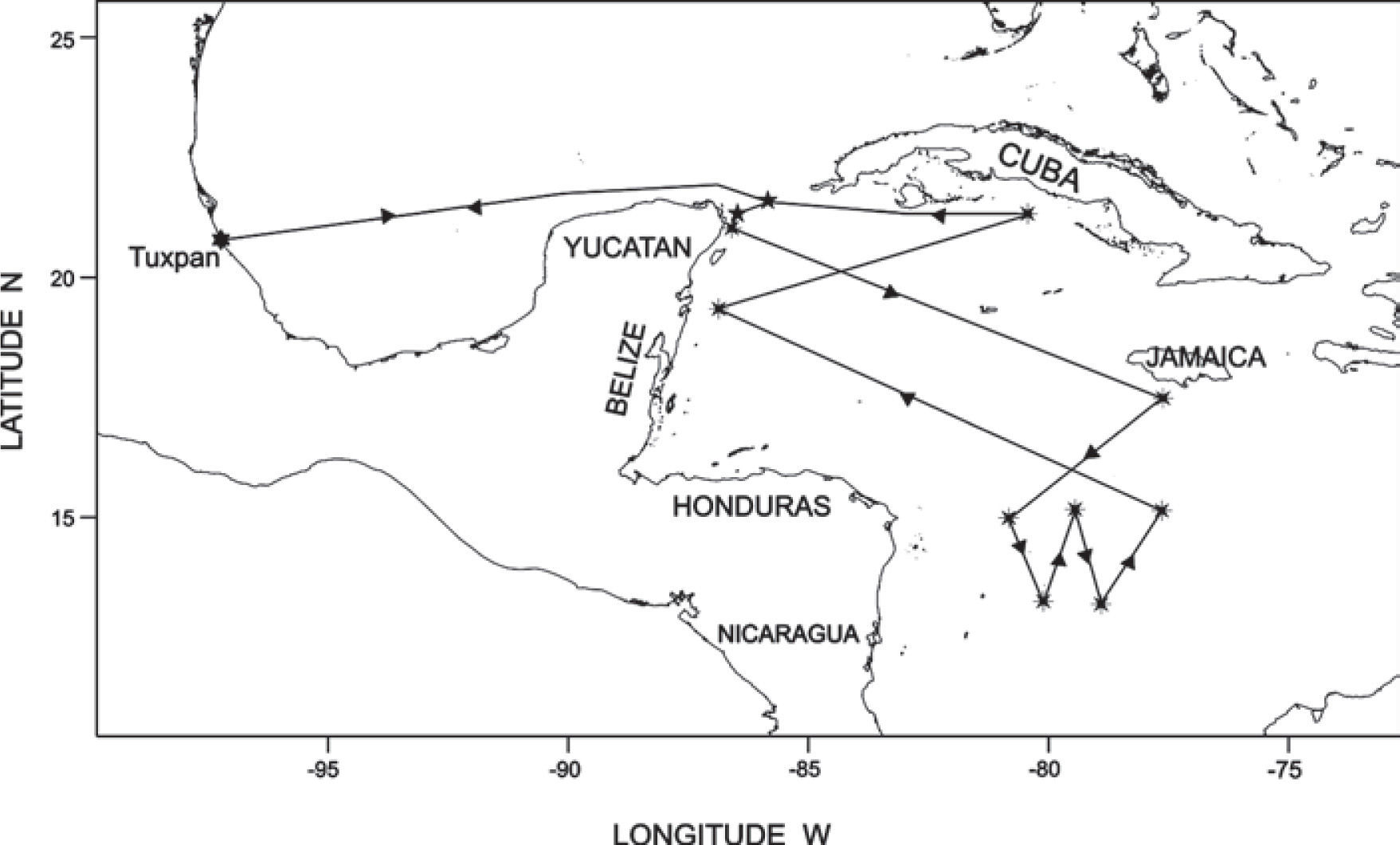
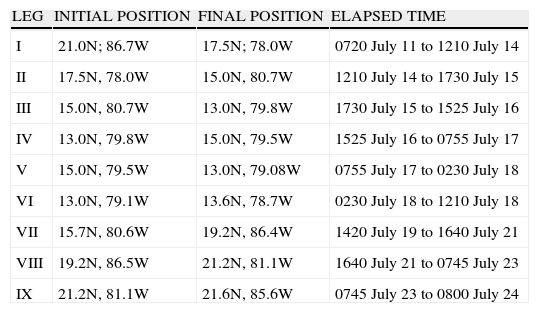
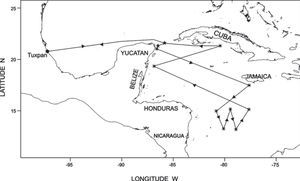
The cruise track had three phases: outbound leg, main survey, and inbound leg (Figure 1). The outbound leg (from Tuxpan to Yucatan channel) was used for testing and training purposes. The cruise track for the main survey was determined by turning points that defined the nine cruise legs (Table I). The inbound leg (from Yucatan Channel to Tuxpan) was used for data processing and analysis, and database mergers.

Figure 1.

ECAC-3 cruise track from July 6 to 26, 2001.

Table 1.

Geographical positions of the nine transects sampled in the Caribbean Sea.

LEG	INITIAL POSITION	FINAL POSITION	ELAPSED TIME
I	21.0N; 86.7W	17.5N; 78.0W	0720 July 11 to 1210 July 14
II	17.5N, 78.0W	15.0N, 80.7W	1210 July 14 to 1730 July 15
III	15.0N, 80.7W	13.0N, 79.8W	1730 July 15 to 1525 July 16
IV	13.0N, 79.8W	15.0N, 79.5W	1525 July 16 to 0755 July 17
V	15.0N, 79.5W	13.0N, 79.08W	0755 July 17 to 0230 July 18
VI	13.0N, 79.1W	13.6N, 78.7W	0230 July 18 to 1210 July 18
VII	15.7N, 80.6W	19.2N, 86.4W	1420 July 19 to 1640 July 21
VIII	19.2N, 86.5W	21.2N, 81.1W	1640 July 21 to 0745 July 23
IX	21.2N, 81.1W	21.6N, 85.6W	0745 July 23 to 0800 July 24

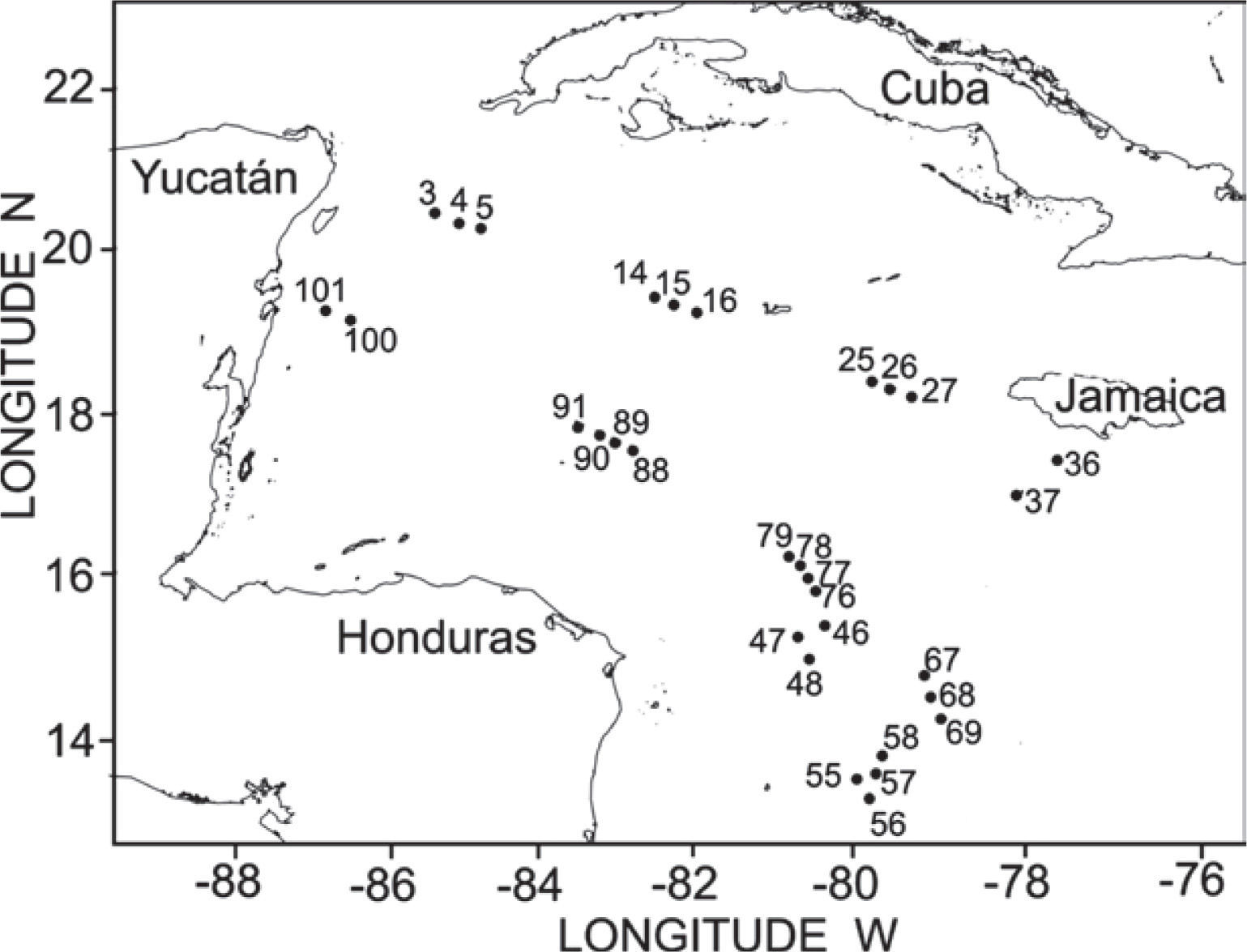
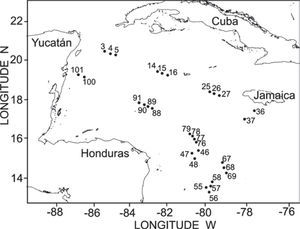
Oceanic observations were focused on CTD casts made four or more times daily for temperature, salinity, density and dissolved oxygen (DO) depth profiles. Daytime fluorescence and reflectance measurements were carried out at 31 sampling stations located along of six cruise legs covering great extension of the Caribbean Sea (Figure 2). Chlorophyll a concentration at the 31 sampling stations was estimated by simultaneous derived SeaWiFs information.

Figure 2.

Location of 31 daytime sampling sites.

Reflectance Measurements

Reflectance was measured by the BDRF method (Swain and Davis, 1978). The BDRF can be defined as:

where r_ti is the flux reflected by a target under specified conditions of irradiation and viewing, r_ci is the flux reflected by a perfectly diffuse, completely reflecting Lambertian surface which is used as a reference and k is the panel correction factor which depends on the angular configuration and accounts for the non-Lambertian spectral reflectance of the panel. To measure r_ti the Spectroradiometer GER-1500 was placed over the sunny side of the ship to avoid the direct shadow of the ship, which may introduce an error of up to 30% (Gordon, 1985). Values of r_ci were obtained by comparison with a Spectralon reference panel (Labsphere). The panel is resistant and hydrophobic, characteristics that render it suitable for field-based research (Schutt et al., 1981; Weidner and Hsia, 1981). The sensor was positioned to provide a nadir view of the water surface, reducing thus the unwanted irradiance of the specularly reflected light, which is independent of the optical properties of the water (Shifrin, 1988). This configuration guaranteed that light backscattered from the sea and the elements present within it would be the major contributor of the signal. Reflectance spectra were obtained by first scanning the water surface and then sequentially measuring over the reference panel. These observations were carried out while trying to keep the same viewing geometry by looking at nadir in both cases. However, since the calibration panel was onboard the target and reference observations were performed at different heights. Then, both scans were divided according to equation (5) and multiplied by a correction factor of k = 0.99, following the manufacturer's instructions.

Spectroradiometric measurements were performed between 9:00 and 15:00 hrs for suitable solar conditions of illumination, that is, 45° around nadir view, as suggested by Jerlov, (1968).

Remote Sensing reflectance spectra were estimated through ad hoc formulas taken a Fresnel reflectance p = 0.028, which minimizes the effects of Sun glint and non-uniform sky radiance, avoids instrument shading problems and is reliable for wind speeds less than 5 m s⁻¹ (Mobley, 1999). Additionally, according to SeaWiFS protocol (Mueller and Austin, 1995) and in order to remove residual surface reflectance due to wave facets, the spectra were corrected for sun/sky glint error by subtracting the reflectance measured at 750 nm from each spectrum.

Derivative Spectral Analysis

Atmospheric effects such as sky radiance, Rayleigh scattering, ozone absorption and Mie (or aerosol) scattering are unwanted signals in marine optics and in ocean colour studies (Sturm, 1981; Gordon, 1978). These effects must be considered in studies using remote sensing reflectance and have to be reduced if not eliminated. Atmospheric normalization algorithms can be used when analysing data for which there are no simultaneous atmospheric measurements. The derivative method is a kind of algorithm which makes insensitive to atmospheric effects some spectral features of the target. Generally, atmospheric normalization algorithms serve to parameterize the spectral shape (slope, curvature, etc) of the remote observations in an attempt to relate them to invariant spectra (Philpot, 1991). Grew (1981), developed a simple empirical algorithm for estimating oceanic chlorophyll from Multichannel Ocean Color Sensor (MOCS) data, the so-called “inflection ratio algorithm”. This algorithm is a linear difference operator acting as a high pass filter. Thus, linear variations in λ are completely removed and high order variations are reduced to a constant. Hence, the filtering quality of derivatives together with is linear properties account for its sensitivity to chlorophyll and its capabilities for eliminating unrelated effects such as the atmospheric ones. In this study a fourth derivative filter was applied to reflectance spectra for locating and identifying ubiquitous peaks concealed in the spectral curves. Since differentiation tends to magnify effects of high-frequency noise in the spectra, a polynomial fit smoothing filter was applied over the data prior to calculation of the derivatives. Smoothed and derivative spectra were obtained with PeakFit program (AISN software, 1995). This program applies a convolution among the reflectance spectra and low and high -pass filters (smoothing and fourth derivative filters, respectively).

Particularly, the fourth derivative detects the presence of both maxima and minima peaks that correspond to reflectance and absorption regions, respectively, due to optical properties of seawater and elements contained within it. Interpretation of peaks was based on the physical and biological spectral properties of these constituents.

Seawifs Data

SeaWiFS data were utilised for associating reflectance and absorption peaks to chlorophyll a concentration. Daily images were not available due to cloud coverage in some of them, thus a set of existing of SeaWiFS imagery during the month was used for estimating the chlorophyll a concentration ([Chl-a]) at each sampling station. A monthly-averaged image, with a pixel of 1 km², was obtained. From this, [Chl-a] was estimated by using the empirical algorithm OC4 (O'Reilly et al, 1998; O'Reilly et al., 2000). In oligotrophic waters, the [Chl-a] and its covarying derived products are considered to be the main contributors to the water reflectance. A simple relationship, with a sigmoid shape, links the log-transformed reflectance ratios or remote sensing reflectance (R_rs) to [Chl-a]. The OC4 algorithm uses a single set of coefficients applied to the blue-green ratio R_BG which is determined by the maximum band ratio approach.

Thus, for R equal to log₁₀(R_BG):

SeaWiFS satellite data were processed with SeaDAS version 6.1. OC4 formulations and coefficients can be obtained from SeaDAS 6.1 documentation.

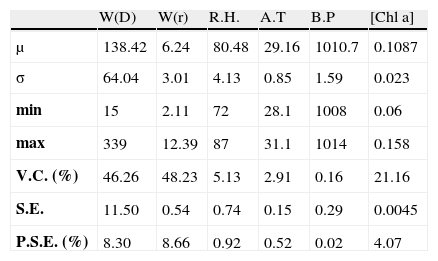
Results and Discussion

Meteorological conditions, measured on board during the cruise, were rather stable except for two days of adverse weather at mid July (Table II). Mean values of the parameters indicate the stability of weather conditions. Thus, wind vectors had a mean magnitude of 6.24(3.01) m s⁻¹ at 138.42(64.04) degrees. Variability of the other meteorological parameters was less: Mean Relative humidity 80.48% (4.13); mean barometric pressure 1010.7 (1.59) mb; and air temperature 29.16 (0.85) (°C).

Table 2.

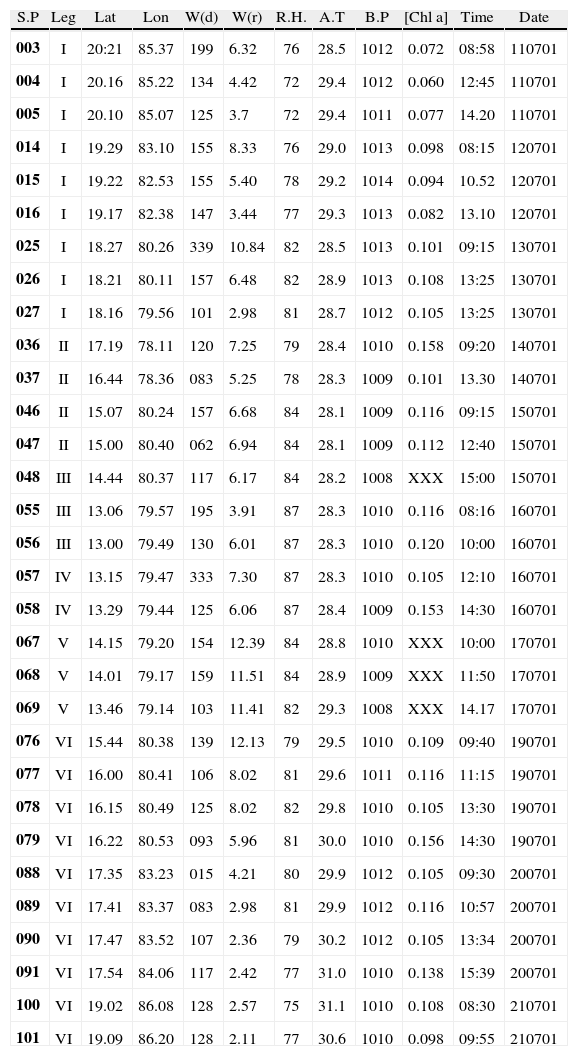
Meteorological and biological parameters measured on board the RV Justo Sierra. S. P. (Sampling point); L (Cruise leg); Lat, (Latitude: °N); Lon, (Longitude: °W); W(d), Wind direction (°); W(r), Wind speed, (m s⁻¹); R. H., relative humidity (%); A.T., Air Temperature, (°C); B. P., barometric pressure, (mb); Chlorophyll concentration [Chl-a] (mg m⁻³); time of reflectance measurements (hh:min); date (ddmmyy).

S.P	Leg	Lat	Lon	W(d)	W(r)	R.H.	A.T	B.P	[Chl a]	Time	Date
003	I	20:21	85.37	199	6.32	76	28.5	1012	0.072	08:58	110701
004	I	20.16	85.22	134	4.42	72	29.4	1012	0.060	12:45	110701
005	I	20.10	85.07	125	3.7	72	29.4	1011	0.077	14.20	110701
014	I	19.29	83.10	155	8.33	76	29.0	1013	0.098	08:15	120701
015	I	19.22	82.53	155	5.40	78	29.2	1014	0.094	10.52	120701
016	I	19.17	82.38	147	3.44	77	29.3	1013	0.082	13.10	120701
025	I	18.27	80.26	339	10.84	82	28.5	1013	0.101	09:15	130701
026	I	18.21	80.11	157	6.48	82	28.9	1013	0.108	13:25	130701
027	I	18.16	79.56	101	2.98	81	28.7	1012	0.105	13:25	130701
036	II	17.19	78.11	120	7.25	79	28.4	1010	0.158	09:20	140701
037	II	16.44	78.36	083	5.25	78	28.3	1009	0.101	13.30	140701
046	II	15.07	80.24	157	6.68	84	28.1	1009	0.116	09:15	150701
047	II	15.00	80.40	062	6.94	84	28.1	1009	0.112	12:40	150701
048	III	14.44	80.37	117	6.17	84	28.2	1008	XXX	15:00	150701
055	III	13.06	79.57	195	3.91	87	28.3	1010	0.116	08:16	160701
056	III	13.00	79.49	130	6.01	87	28.3	1010	0.120	10:00	160701
057	IV	13.15	79.47	333	7.30	87	28.3	1010	0.105	12:10	160701
058	IV	13.29	79.44	125	6.06	87	28.4	1009	0.153	14:30	160701
067	V	14.15	79.20	154	12.39	84	28.8	1010	XXX	10:00	170701
068	V	14.01	79.17	159	11.51	84	28.9	1009	XXX	11:50	170701
069	V	13.46	79.14	103	11.41	82	29.3	1008	XXX	14.17	170701
076	VI	15.44	80.38	139	12.13	79	29.5	1010	0.109	09:40	190701
077	VI	16.00	80.41	106	8.02	81	29.6	1011	0.116	11:15	190701
078	VI	16.15	80.49	125	8.02	82	29.8	1010	0.105	13:30	190701
079	VI	16.22	80.53	093	5.96	81	30.0	1010	0.156	14:30	190701
088	VI	17.35	83.23	015	4.21	80	29.9	1012	0.105	09:30	200701
089	VI	17.41	83.37	083	2.98	81	29.9	1012	0.116	10:57	200701
090	VI	17.47	83.52	107	2.36	79	30.2	1012	0.105	13:34	200701
091	VI	17.54	84.06	117	2.42	77	31.0	1010	0.138	15:39	200701
100	VI	19.02	86.08	128	2.57	75	31.1	1010	0.108	08:30	210701
101	VI	19.09	86.20	128	2.11	77	30.6	1010	0.098	09:55	210701

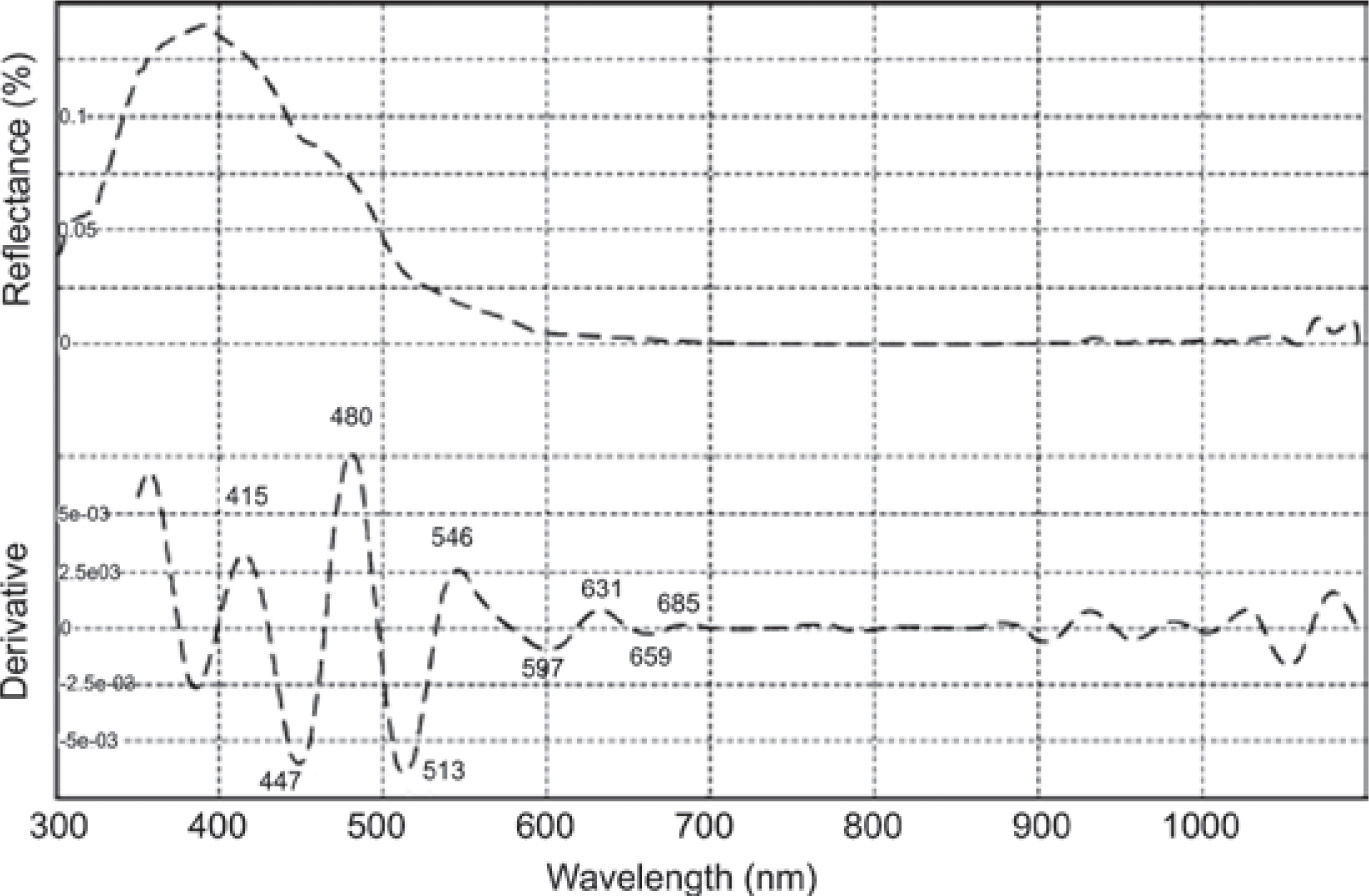
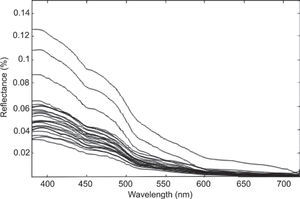
The spectral response of the Caribbean Sea is rather homogeneous. Most spectra profiles had a similar trend, with a relatively high reflectance between 0.04 and 0.06 at the blue end (400–500 nm), except for three cases showing higher values; an abrupt downward slope starting at around 500 nm due to water absorption, and lower values at the red end of the spectra (Figure 3). All spectra are modulated by the optical properties of oceanic water and, at a lesser extent, by phytoplankton, which is characteristic of oligotrophic conditions. These characteristics account for the typical blue coloration of Caribbean waters.

Figure 3.

Reflectance profiles of 31 daytime sampling stations.

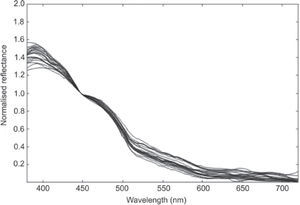
The derivative analysis provides a more accurate location of absorption and reflectance peaks. They slightly differ from analysis performed with conventional methods. The derivative method precisely locates and the actual inflexion points and, consequently, identifies absorption or reflection peaks. Thus, a fourth derivative filter was then applied to all spectra in order to detect hidden reflectance and absorption peaks. Each reflectance spectra showed similar reflectance and absorption peaks as maxima and minima values, respectively. Hence, instead of having the theoretical blue absorption peak of chlorophyll around 440 nm, with the derivative analysis this peak is found around 447 nm. A statistical analysis of all the peaks found through this method was performed for the reflectance curves of every sampling site. Consequently, a mean value of them was considered in the following derivative analysis (Table IV). When all reflectance spectra were normalised at 447.95 (mean value of the fourth derivative maxima), which corresponds to the absorption peak of chlorophyll a in the blue region, the differences and similarities among the spectra could be assessed (Figure 4). Major differences are apparent between 400 and 450 nm (blue), whilst similarities occur mainly in the 450 – 500 nm region (green). From 500 nm onwards all spectra have the same trend with slight variations between 650 and 700 nm.

Table 4.

Reflection (R_i) and Absorption (A_i) peaks found through derivative analysis.

S.P.	R1	R2	R3	R4	R5	A1	A2	A3	A4
003	414.92	479.01	544.94	637.62	696.50	446.06	512.01	605.35	666.41
004	418.18	480.66	544.94	624.75	680.70	449.35	513.66	595.60	652.05
005	414.92	482.31	546.58	636.02	698.08	447.7	512.01	602.11	668.30
014	411.65	479.01	546.58	631.19	688.61	447.7	512.01	600.48	660.03
015	416.55	480.66	544.94	627.97	680.70	449.30	512.01	597.23	653.65
016	419.82	480.66	544.94	621.52	675.94	449.35	512.01	592.36	647.25
025	411.65	480.66	546.58	634.41	691.77	446.06	512.01	598.86	664.81
026	413.29	479.01	544.94	627.97	683.86	446.06	512.01	595.61	653.65
027	418.80	480.66	544.94	626.36	675.94	447.70	513.66	595.60	652.05
036	406.77	480.66	546.58	634.41	691.70	447.7	512.01	592.36	659.59
037	419.82	474.06	543.29	640.83	679.11	442.77	512.01	559.70	663.22
046	414.92	479.01	548.22	632.80	690.19	447.70	513.66	598.86	663.22
047	418.18	480.66	544.94	634.40	680.70	447.70	513.66	606.97	660.03
048	411.65	480.66	548.22	632.80	694.92	447.70	513.66	600.48	661.63
055	414.92	480.66	558.06	631.19	691.77	449.35	515.31	600.48	660.03
056	413.29	482.31	546.58	627.97	671.18	447.70	512.01	597.23	652.05
057	419.82	480.66	541.65	645.64	691.79	449.35	512.01	558.06	647.25
058	413.29	480.66	546.58	627.58	675.94	447.70	512.01	595.61	652.05
067	411.65	480.66	548.22	632.80	691.77	449.35	513.66	603.73	663.22
068	408.40	482.31	559.70	642.44	696.50	452.64	515.31	597.23	672.77
069	414.92	480.66	546.58	629.58	680.70	447.70	513.66	600.48	656.84
076	414.92	480.66	551.30	632.80	688.81	447.70	513.66	600.48	663.22
077	414.92	482.31	546.58	619.91	672.77	449.35	515.31	592.36	645.64
078	416.55	480.66	544.94	629.58	682.28	447.70	513.66	602.11	655.24
079	410.02	480.66	546.58	623.14	671.18	449.35	513.66	597.23	645.64
089	418.18	480.66	544.94	627.97	682.28	447.70	512.01	600.48	655.24
100	414.92	479.01	544.94	632.80	693.35	449.35	512.01	602.11	661.63
101	413.29	479.01	546.48	632.80	694.92	447.70	512.01	600.48	664.81
102	418.18	480.66	544.94	626.36	675.94	447.70	513.66	600.48	652.05
103	421.45	479.01	548.22	644.04	694.92	447.70	513.66	600.48	671.18
112	414.92	479.01	546.58	631.19	685.45	447.70	513.66	600.48	660.03
113	418.18	480.66	546.58	639.23	694.92	446.06	513.66	611.83	669.59
114	413.29	482.31	546.58	632.80	687.03	447.70	512.01	602.11	663.22
m	415.04	480.31	546.92	631.90	685.82	447.95	513.01	597.12	659.02
s	03.43	1.54	3.51	6.09	8.19	1.60	1.09	10.67	7.59

Figure 4.

Reflectance spectra normalized at 447.98 nm, corresponding to the region of maximum chlorophyll a absorption.

However, it has been reported the presence also of cyanobacteria and green algae groups (Bidigare et al., 1993). Mean absorption peaks at 597.12 and 659.02 nm are due to pure seawater. There is evidence that light absorption by pure water depends on its temperature, being apparent in absorption bands around 600 and 660 nm at 21 °C (Pegau and Zaneveld, 1993). It has been found that the absorption maximum shifts towards lower wavelengths at increasing temperature (Hakvoort, 1994). This may account for the slight difference found between the two sets of data, since the sea surface temperature of Caribbean waters was around 29 °C during the sampling.

A typical reflectance spectrum with its correspondent derivative peaks is shown in Figure 5. Peaks found through the derivative method can be associated with the optical properties of seawater. Mean reflectance peaks at 415.04 and 480.31 nm stands for the dominant colour of the water, which was mostly bluish over the entire zone. The peak at 685.82 nm is mainly due to the sum of irradiances produced through natural fluorescence emission by chlorophyll a and elastic scattering (Kirk, 2011). The mean reflectance peak at 546.92 nm has a physical explanation rather than a biological one because this peak marks the starting point of the strong water absorption, which reaches a maximum around 600 nm (Buiteveld et al, 1994).

Figure 5.

Typical reflectance profile with its derivative spectrum showing main reflectance and absorption peaks.

On the other hand, absorption peaks found by the derivative process can be essentially related to optical properties of pigments and seawater (Prézelin and Bóczar, 1986; Aguirre et al, 2001). Thus, the peak at 447.95 nm is due to chlorophyll a absorption in the blue region. A carotenoid absorption peak at 513.01 nm can presumably be attributed to fucoxanthin-like pigments of brown algae, which are among the dominant groups in the zone (Taylor, 1959; Müller-Karger et al, 1989; Bidigare et al, 1993).

Satellite-estimated [Chl-a] at each sampling station is shown in Table II and had a mean value of 0.109 (0.023) mg m⁻³ (Table III). Values obtained are typical of oligotrophic waters, mainly at the northern sampling stations (3, 4, 5, 14 and 15), the remaining sites showed a slight increment of [Chl-a] between 0.101 – 0.158 mg m⁻³ but still in the oligotrophic interval, according to the CZCS-NASA climatological monthly maps (Feldman et al., 1989) as seen in Table II and Figure 2. Regions with the highest [Chl-a] values were found close to Jamaica island (sites 25, 26, 27, 36) and near Honduras (sites 58, 79 and 91).

Table 3.

Descriptive statistics (μ, mean: σ: standard deviation; min: minimum value; max: maximum value; V.C. Variation Coefficient; S.E. Standard error; P.S.E.: Percentual Standard error. W(d), Wind direction (°); W(r), Wind speed, (m s⁻¹); R. H., relative humidity (%); A.T., Air Temperature, (°C); B. P., barometric pressure, (mb); [Chl-a] Chlorophyll concentration (mg m⁻³)

	W(D)	W(r)	R.H.	A.T	B.P	[Chl a]
μ	138.42	6.24	80.48	29.16	1010.7	0.1087
σ	64.04	3.01	4.13	0.85	1.59	0.023
min	15	2.11	72	28.1	1008	0.06
max	339	12.39	87	31.1	1014	0.158
V.C. (%)	46.26	48.23	5.13	2.91	0.16	21.16
S.E.	11.50	0.54	0.74	0.15	0.29	0.0045
P.S.E. (%)	8.30	8.66	0.92	0.52	0.02	4.07

Moreover, applying OC4 algorithm to SeaDAS data tends to over-estimates the chlorophyll concentrations in relatively chlorophyll-poor waters, where the relative part of the suspended matter and the CDOM in the optical properties of water is high. For such waters, benefit in accuracy by using one algorithm or the other seems negligible compared with the biases and the noises. However, we have chosen the OC4 band ratio as it is recommended in the literature for a larger variety of waters, including chlorophyll rich waters.

Conclusions

Reflectance characteristics of the Caribbean Sea were analysed with a high spectral resolution radiometer. Reflectance spectra show a typical coloration of tropical waters with maxima at the blue region of the electromagnetic spectrum. This response is mainly due to the oligotrophic characteristics of the zone, with a deep chlorophyll maximum at around 107 m. The presence of chlorophyll a can be established by two ways: a) via fluorescence, by observing the spectral behaviour which has an absorption peak at 447.9 nm (blue absorption) and a reflectance peak at 685.82 nm (fluorescence emission); b) via satellite derived information. Satellite information confirms both the homogenous distribution of [Chl-a] and the oligotrophic condition of the Caribbean Sea. Reflectance profiles are markedly influenced by seawater spectral properties from 600 nm onwards. Hence, even though the Caribbean Sea is oligotrophic, the derivative analysis detects the presence of algae in an amount sufficient to generate a relatively strong signal within reflectance spectra and satellite imagery. Additionally, it can be concluded that most Caribbean Sea waters show similar optical characteristics in open ocean, being higher close to Jamaica and Honduras. This paper contributes to a better understanding of the optical characteristics of the Caribbean Sea.

Acknowledgements

I thank the following: Dr. A. Gallegos for his kind invitation to participate in the ECAC-3 cruise; Dr. V. Magaña, coordinator of the project and the IAI for funding these campaigns; to Dr. Olivia Salmeron and Tania Fernández for support during the cruise; the technicians of the Institute of Geography for drawings; and the crew of RV Justo Sierra.