To evaluate the intraobserver and interobserver reliability of radial torsion angle measurement using computed tomography.
METHODS:Twelve pairs of cadaver radii and 116 forearms from 58 healthy volunteers were evaluated using axial computed tomography sections measured at the level of the bicipital tuberosity and the subchondral region of the radius. During digital imaging, the angle was formed by two lines, one diametrically perpendicular to the radial tubercle and the other tangential to the volar rim of the distal joint surface. Measurements were performed twice each by three observers.
RESULTS:In cadaveric bones, the mean radial torsion angle was 1.48° (-6° - 9°) on the right and 1.62° (-6° - 8°) on the left, with a mean difference between the right and left sides of 1.61° (0° - 8°). In volunteers, the mean radial torsion angle was 3.00° (-17° - 17°) on the right and 2.91° (-16°- 15°) on the left, with a mean difference between the sides of 1.58° (0° - 7°). There was no significant difference between each side. The interobserver correlation coefficient for the cadaver radii measurements was 0.88 (0.72 - 0.96) and 0.81 (0.58 - 0.93) for the right and left radius, respectively, while for the volunteers, the difference was 0.84 (0.77 – 0.90) and 0.83 (0.75 – 0.89), respectively. Intraobserver reliability was high.
CONCLUSION:The described method is reproducible and applicable even when the radial tubercle has a rounded contour.
Pathological or traumatic processes that cause rotational changes to the forearm bones, particularly the radius, may result in persistent pain, limitation of motion and instability at the distal radioulnar joint.1,2 This occurs with malunited fractures that usually result in limited forearm rotation and can lead to degeneration and arthrosis of the wrist and elbow2,3 as well as articular dislocations and subluxations.4
A precise measurement of the radial torsion angle may assist in the proper treatment of fractures and when performing a precise surgical correction for a possible malunion. Measuring rotational deviation is a difficult task, regardless of the method employed. In 1945, Evans proposed comparing radiographs between the fractured and contralateral forearm in standardized positions by focusing on the proximal portion of the radius, where the shape of the radial tubercle could be identified. By comparing radiographs of the two bones, it was possible to determine the approximate rotation of the fractured radius. The distal segment was then positioned according to the position of the proximal segment.5 However, this method requires various radiographic expositions and does not permit precise evaluations or standardization.
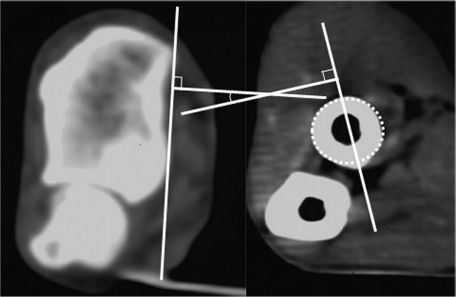
More recently, Bindra et al. described a computed tomography method for measuring rotation based on a measurement of the angle formed by an axis traced tangentially to the radial tubercle on the proximal metaphysis of the radius and another axis traced along the largest width along the distal radius.6 The tomographic sections containing the traced axes are then superimposed, and the angle between them is measured (Figure 1). This method is similar to that used to measure the torsion angle of the tibia and femoral head and has the advantages of low doses of ionizing radiation, accuracy and, allegedly, easy reproducibility.6
However, our personal experience when attempting to reproduce the method of Bindra et al. was that it is not always easy to trace the axis tangential to the radial tubercle because the flattened medial surface of the tubercle on which the biceps tendon rests is not always sufficiently flat to permit the exact apposition of the proximal axis without outward or inward deviation.
The objective of the present study was to present a modification of the method of Bindra et al. in order to develop an alternative technique for measuring the radial torsion angle by means of computed tomography. In addition, we evaluated the interobserver and intraobserver reliability of measurements obtained using this new method.
MATERIAL AND METHODSThis study was approved by the Institutional Research Ethics Committee at our University Hospital (Protocol 344/2007).
First, radial torsion angles were obtained in 12 pairs of denuded radii (Figure 2) selected from skeletons stored in the Forensic Medicine Institute affiliated with the Medical School. The selected bones were well preserved, including a fully preserved bone structure. The bones were made available for this study with the caveat that they would be returned without any violation of their physical integrity.
In the second step, the authors studied the radial torsion angles of both the right and left radii of 58 healthy volunteers (Table 1). All subjects signed a written informed consent to participate and answered a questionnaire with information about gender, age, hand dominance and their profession. Only volunteers without a history of previous fractures of the upper limbs were included. Thirty-two of the 58 volunteers were men (55.17%), and 26 were women (44.94%), resulting in a mean age of 34.18 years (range: 19-77 years). In addition, 51 were right handed (87.93%). The study population was distributed into six age ranges. Most volunteers (74.12%) were in the 21 to 40 year age range, with a high prevalence (N = 31, 53.44%) of subjects aged 21 to 30 years.
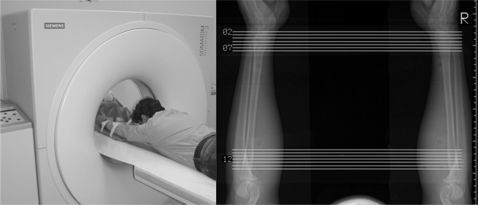
All tomographic exams were performed with the same equipment (SIEMENS, SOMATON Emotion model, Forchheim, Germany). The volunteers were positioned in the prone decubitus position on the exam table with both upper limbs positioned with maximal extension of shoulder and with the forearms attached by Velcro straps to a wooden positioning device and with the hands clasping vertically positioned pins (Figure 3). The images were obtained in the transverse plane of the forearm with 120 kV, 160 mA and 1 second of rotation. Along the proximal third of the radius, the sections included a 2 cm segment of bone centered on the radial tubercle. Distally, images were obtained at the epiphysis and included the subchondral bone of the distal articular surface.6 The radial torsion angle was measured using the E-film program, version 2.1.1.
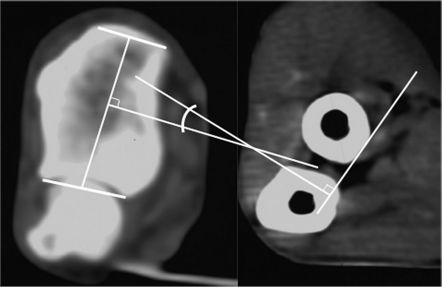
A reference line (line A) was traced on the volar rim of the distal radius, as recommended by Dumont et al.7 Another line, called line B, was traced on a diametrical path perpendicular to the most prominent point of the radial tubercle on the proximal radius (Figure 4). The diameter was related to the best circumference that fit the radius cross section; the insertion of the biceps tendon was visible within the soft tissue window and served as a reference that helped to find the proper point on the radial tubercle.
An alternative method for measuring the radial torsion angle with a distal line drawn tangential to the volar edge of the radius on the left side and a proximal line drawn diametrically to the radial tubercle on the right side. The biceps tendon insertion helped localize the most prominent point of the radial tuberosity.
The radial torsion angle was measured twice and independently by three different examiners with a one-week interval between measurements. Two of the examiners were fully licensed, experienced orthopedic surgeons; the third examiner was a 2nd year orthopedic resident.
Each examiner selected the most adequate tomographic section, recognized the predefined parameters, drew the appropriate lines, superimposed the sections and measured the angles independently of the primary examiner. A mean value was calculated for the two measurements for each radius and each examiner, and the difference between the two sides for both the denuded bones and the volunteers was also calculated. Finally, a general mean value was calculated for each side as well as for the difference between sides.
Data underwent inter- and intraexaminer statistical analysis using a nonparametric Wilcoxon test and nonparametric Spearman correlation to determine the degree of correlation between measurements. Data for the volunteers (mean values for the right and left sides and difference between them) were also analyzed with regard to gender, hand dominance and age using a nonparametric Mann-Whitney test for the first two items and a non-parametric Kruskal-Wallis test for the last item. A nonparametric Wilcoxon test for paired samples was used to compare the measurements obtained on each side in each exam by each observer. The correlation between the inter- and intraobserver values was also determined with a nonparametric Spearman correlation test. The confidence interval adopted was 95% (p<0.05).
RESULTSThe overall results are illustrated and summarized in Tables 2 through 5. Differences between the right and left radii were not statistically significant for either the denuded bones (p = 0.62) or for volunteers (p = 0.58). There was also no significant difference between gender (p = 0.96 and p = 0.85 for the right and left radius, respectively) or hand dominance (p = 0.72 and p = 0.90 for the right radius and left radius, respectively). No significant difference was found between measurements in the cadaveric bones and in volunteers (p = 0.08). There was also no correlation between age and the radial torsion angle (p = 0.73 and p = 0.76 for the right and left radius, respectively).
In denuded bones, the mean radial torsion angle (RTA) was 1.48° (-6° - 9°) on the right and 1.62° (-6 ° - 8°) on the left, with a mean difference between the right and left sides of 1.61° (0° - 8°). In volunteers, the mean RTA was 3.00° (-17° - 17°) on the right and 2.91° (-16°- 15°) on the left, with a mean difference between sides of 1.58° (0° - 7°).
The interobserver correlation coefficient for the measurements performed in the denuded bones was 0.84 (range: 0.77 – 0.90) and 0.83 (range: 0.75 – 0.89) for the right and left radius, respectively. For volunteers, the interobserver correlation was 0.84 (0.77 - 0.90) and 0.83 (0.75 - 0.89) for the right and left radius, respectively.
The intra-observer correlation coefficient between the first and the second measurement for the first observer, who was less experienced, was 0.65 and 0.28 for the right and left radius, respectively. For the second examiner, these values were 0.85 and 0.82 for the right and left radius, respectively, and for the third examiner, these values were 0.91 and 0.88 for the right and left radius, respectively.
DISCUSSIONThere is a close correlation between bone shape and function. As a result, the primary objective of fracture treatment should be the restoration of anatomy to permit the preservation of range of motion. A frequent complication of radius fractures is malunion associated with angular changes, rotational changes and shortening, which almost always results from inadequate conservative treatment. With surgical treatment, angular changes and shortening can be fully corrected with an open reduction and internal fixation. Nevertheless, a rotational deviation may persist, particularly in the case of multi-fragmented fractures where the deformity may be difficult to detect by direct visualization. The persistence of rotational deviation almost always reduces the amount of forearm rotation around its longitudinal axis and may result in an important blockade of pronation or supination.
Angular deformities of the forearm bones are easily diagnosed both clinically and radiologically, in contrast to rotational deformities, which are frequently underestimated.2,8 Identifying the origin of a forearm rotational deficit may be difficult due to the lack of any accurate and reproducible method that has been validated in population studies. The existence of such a method would permit more faithful surgical planning and adequate correction by osteotomy.
Methods previously proposed to quantify rotational deviations include serial Evans radiographs, the method of Bindra et al., magnetic resonance imaging and fluoroscopy with a goniometer. All have drawbacks ranging from a high exposure of ionizing radiation to the relatively high costs associated with MRI. In addition, none of the existing methods is sufficiently accurate to identify rotational deviations of the radius of less than 35°. However, rotational deviations less than 35° have been described as important in the reduction of forearm rotation.7,9-12
The study by Bindra et al. is based on computed tomography measurements and is potentially precise; it also has the advantage of limited ionizing radiation because the exam focuses exclusively on the proximal and distal ends of the radius over a short segment, implying a relatively low cost.6,11 However, the application of this method at our institution revealed significant difficulty in properly identifying the plane contiguous to the radial tubercle because 33.3% of our cadaveric radii and 60.34% of volunteer radii had a radial tubercle with rounded contours on axial sections. This may reflect a racial or ethnic difference between populations.
We observed in this series that it was possible to consistently identify the most elevated point of the radial tubercle by passing a line through it on a diametral path (Figure 4). At the distal end of the radius, we also found that it was easy to draw a line along the palmar margin of the distal articular surface.
Previous studies have shown a wide variation in the absolute values of the radial torsion angle, resulting in a very wide range of normal values.6,7 The parameters adopted in the present study for the measurement of the radial torsion angle differed from those employed in other studies, resulting in considerably different absolute values compared to previously reported data but a substantially lower variation between sides for both denuded bones and volunteers. The highest variation in absolute values for the right and left denuded bones was 8°, with a mean radial torsion angle of 1.48° on the right and 1.62° on the left.
Some authors have stated that the most important and reliable finding for an evaluation is the difference between sides.6,7 Former investigators have obtained a mean difference between sides of 4.9°, with a range from 0.01° to 18.1° 6. In another study a maximum difference of 30° between sides was found using a method based on nuclear magnetic resonance and a maximum difference of 34.5° was obtained using the method of Bindra et al.7 They concluded that the diagnosis of a malunited fracture should be made only if the difference between sides was more than 35°, which was an indication of a corrective osteotomy.
In the present study, the mean difference between the right and left radii was 1.61°, with a considerably smaller range (that is, 0° to 8°). Following the same line of reasoning as former investigators, this method would diagnose a malunited fracture with a difference of more than 8°; the need for a corrective osteotomy would depend on other factors.
Variables such as gender, age and hand dominance were assessed and correlated with radius angulation. The differences between the right and left radii were significantly greater among women than among men (p = 0.03). However, there was no significant difference between men and women with respect to measurements of the right and left radii.
Neither age nor hand dominance seemed to modify the radial torsion angle in any significant way. Furthermore, the differences across age ranges and between the right and left radii were insignificant. Thus, in either case, the method reported here can be applied without fear of erring above or below normal values. There was high interobserver consistency for all the observers and high intraobserver reliability for the two more experienced observers.
CONCLUSIONThe method described here for the measurement of the radial torsion angle using computed tomography is an alternative to the method proposed by Bindra et al. In addition, the method presented here is easy to execute and reproducible across observers; thus, it has the potential for clinical application in the evaluation of rotational deformities of the radius.