Cognitive performance and academic achievement: How do family and school converge?

Abstract

Children enter the school system with different educational experiences, leaving also with different levels of learning and school results. In this study, we intend to understand the impact of family and school on children's cognitive performance and academic achievement during elementary education. The sample consists of 406 Portuguese children, from preschool and the 1st cycle of Basic Education, aged from 4 to 10 years old. Through full structural equation model, it was observed that the latent variable family (parents’ schooling and socioeconomic level) and the latent variable school (community and type of school) have a significant impact on academic achievement. However, only family presents a significant impact on cognitive performance. These data suggest that the impact of school on intelligence quotient is not expressive in early academic years, where family present higher explanation of the variance.

Resumen

Los niños ingresan en el Sistema Educativo con diferentes experiencias escolares, alcanzando también diferentes niveles de aprendizaje y resultados escolares. En este estudio, nuestra pretensión es comprender el impacto de la familia y de la escuela en el desempeño cognitivo y el rendimiento académico de los niños durante la educación primaria. La muestra se compone de 406 niños portugueses de preescolar y del 1° ciclo de Educación Primaria, con edades comprendidas entre los 4 y los 10 años. Por medio de modelo completo de ecuaciones estructurales, se observa que la variable latente familia (nivel de escolaridad y nivel socioeconómico) y la variable latente escuela (comunidad y tipo de escuela) tienen un impacto significativo en el rendimiento académico. No obstante, solo la familia presenta un impacto significativo en el desempeño cognitivo. Estos resultados sugieren que el impacto de la escuela en el cociente de inteligencia no es tan relevante en los primeros años o niveles escolares, donde la familia presenta mayor explicación de la varianza.

Introduction

The concept of intelligence (structure and evaluation) is still debated among experts; however, it gathers some consensus in research as a significant predictor of quality of learning (Almeida, Guisande Primi, & Lemos, 2008; Spinath, Spinath, Harlaar, & Plomin, 2006; Sternberg, 2012; Sternberg, Grigorenko, & Bundy, 2001; Strenze, 2007) and is considered, par excellence, a variable that differentiates levels of academic achievement (Deary, Strand, Smith, & Fernandes, 2007; Lemos, Almeida, & Colom, 2011; Primi, Ferrão, & Almeida, 2010). However, this relationship is not stable over time, as there is a gradual decrease of correlation coefficients as schooling advances, suggesting the importance of other variables, namely socio-familial (Freitas, Simões, Alves, & Santana, 2012; Nisbett et al., 2012).

Studies on cognitive development and on teaching and learning processes refer to a significant relationship between family involvement and academic success of children, and consequently to their school trajectory (Álvarez et al., 2015; Alves, Gomes, Martins, & Almeida, 2016; Eslava, Deaño, Alfonso, Conde, & García-Señorán, 2016; Fernández-Zabala, Goñi, Camino, & Zulaika, 2016; Kloosterman, Notten, Tolsma, & Kraaykamp, 2011; Lugo-Gil & Tamis-LeMonda, 2008; Mistry, Benner, Biesanz, Clark, & Howes, 2010; Regueiro et al., 2015). In this regard, the level of parental education stands out, which seems to differentiate educational strategies and how parent and child interact (Bracken & Fischel, 2008; Eslava et al., 2016; Martínez, Martínez, & Pérez, 2004; Oxford & Lee, 2011; Rindermann, Flores-Mendoza, & Mansur-Alves, 2010). Recent investigations indicate that the mother's academic qualifications emerge as a better predictor of cognitive performance (Gutman, Sameroff, & Cole, 2003). Studies of the Senna project in Brazil have shown the impact of socio-familial variables on children's academic motivation (Santos & Primi, 2014). Still, within the family, there is evidence that the family's socioeconomic status influences the academic performance of children, with the most prominent influence being in childhood (Alves et al., 2016; Rindermann et al., 2010). Studies have shown that higher socioeconomic conditions are associated with better cognitive performance of children, since such conditions allow greater access to spaces and playful, educational and cultural materials (Alves et al., 2016; Bradley & Corwyn, 2002; Burger, 2010; Cabrera, Shannon, & Tamis-LeMonda, 2007; Lemos et al., 2011; Strenze, 2007). On other hand, lack of assets and an overcrowded house can limit the availability of educational resources for children, having a negative effect on later school learning processes (Ainscow et al., 2010; Downey, 2001; Goux & Maurin, 2005).

One topic in the debate is the role of school in the cognitive development of children and in overcoming cognitive difficulties. Some authors state that children from families with lower socioeconomic and cultural resources experience greater school difficulties, facing school with a more limited vocabulary and numeracy skills. These difficulties and discrepancies that benefit children from the most favored social strata tend to persist during subsequent school years (Burger, 2010; Magnuson, Meyers, Ruhm, & Waldfogel, 2004), especially if early interventions to overcome the same difficulties are not implemented (Burger, 2010). This notion of the stability of school difficulties refers us to the reproductive role of the school, evidencing that social inequalities turn into school inequalities that, in turn, perpetuate the former inequalities. Sullivan (2001) adds that school can contribute to social reproduction (according to Bourdieu's theory of cultural and social reproduction), considering that school curricula value abilities very much associated with the cultural capital of the higher social classes. However, recently, studies have shown that schooling can lessen the impact of deprivation on children's progress. Nevertheless, school systems have no control over some factors that limit their impact (Ainscow et al., 2010). It is likely that the impact of schooling will occur in the form of modest improvements for disadvantaged children, rather than essential life modifications. Even so, there is reason for optimism, as these possible positive impacts are worth the effort (Ainscow et al., 2010).

In recent decades, school emerges as a privileged context for the psychosocial development of the young people, in particular for their cognitive and learning development. Thus, society and families believe and invest in it at progressively younger ages, and it is assumed that its impact on children's cognitive, social and affective development grows as their schooling increases (Ceci, 1991; Cliffordson & Gustafsson, 2008; Gustafsson, 2001; Stelzl, Merz, Ehlers, & Remer, 1995). This variable “school effect” (Coleman, 1966) is crucial in the case of promoting success among students from more disadvantaged socio-cultural backgrounds. When comparing schools and levels of learning/outcomes for the most disadvantaged students, the school they attend has a significant weight in academic achievement (Heyneman, 1986). The same author stresses that the quality of the school and its teachers is one of the most decisive factors for learning. In a meta-analysis, 28 factors that influence learning were identified, where the teacher emerges as the factor with the greatest influence on student learning, even ahead of family variables (Wang, Heartel, & Walberg, 1994). In this line of thought, a study of the effects of changes in length of schooling in six European countries found that longer compulsory education improves cognitive performance up to four decades later (Schneeweis, Skirbekk, & Winter-Ebmer, 2014). It should be noted that community (rural vs. urban) and type of school (public vs. private) are also relevant in cognitive performance and academic achievement. Generally, urban children and children attending private schools have better results (Strenze, 2007; Wechsler, 2003). This superiority has been explained by greater accessibility to diversified cultural spaces and more enriched pedagogical activities (Veiga, Galvão, Festas, & Taveira, 2012). Throughout recent decades, several studies have analyzed the determinants of academic achievement; that is, the factors that interfere in children's learning and school performance, highlighting the convergence of personal, family, and school factors (Lee & Shute, 2010; Winne & Nesbit, 2010). At the same time, and supporting the impact of schooling, it is assumed that previous school performance is determinant of the level of subsequent school performance in future grades (Hailikari, Nevgi, & Komulainen, 2008; Soares, Lemos, Primi, & Almeida, 2015). This study analyzes the impact of two latent variables concerning family and school dimensions on cognitive performance and academic achievement. Cognitive performance was estimated by the scores in an intelligence tests battery and the academic achievement was calculated taking the teachers classifications. Finally, we analyze the relationship between children's intelligence tests score and the academic achievement. A sequence of tree hypothesis has been tested in the model: (1) family and school have impact on children's cognitive performance; (2) family and school impact on children's academic achievement; (3) with increased schooling, school has more impact on intelligence quotient and academic achievement.

Method

Participants

In Portugal, compulsory education is organized into three levels (1st, 2nd and 3rd) of basic education, with nine grades, and a secondary education level having three grades. Our study includes children attending the last year of pre-school (year before compulsory schooling) and attending the first cycle of basic education (including four school years). The sample is composed of 406 Portuguese children attending preschool education (N = 150) and 1st cycle of basic education (N = 256) (2nd and 4th grade, N = 123 and N = 133 respectively), aged between 4 and 10 years old (M = 6.91, SD = 1.82), equally distributed by gender (50.0% boys and 50.0% girls), living in rural (48.0%) and urban (52.0%) areas in northern and central region of the country, attending public (67.7%) and private (32.3%) institutions participated in this study. Children identified with special educational needs and having experienced grade retention in school were not considered in the study in order to control other academic variables (less than 5% of children in these situations).

Measures

The Cognitive Skills Scale for Children from 4 to 10 years old – ECCOs 4/10 is a cognitive assessment battery of individual application, created for the Portuguese population (Brito & Almeida, 2009). ECCOs 4/10 organizes subtests into a sequence of six cognitive operations that assess the perception (codification and perceptual attention to details), short-term memory (attention, retention and immediate recall of digits), understanding (grasping elements and senses in a context), reasoning (grasping and application of relations between elements), problem-solving (performing tasks guided by a broader scope of information to be processed) and divergent thinking (production of ideas, originality and fluency) (Brito & Almeida, 2009). These six processes are evaluated through tasks using two types of content: one connected to verbal tasks, while the other is more figurative, manipulative and practical, maintaining a similar structure of traditional Wechsler's intelligence scales for children (WISC, WPPSI). The results obtained with this scale show high internal consistency indices, between .87 and .97. Validity studies show a significant and positive correlation between ECCOs and WPPSI or WISC scores (Brito & Almeida, 2009; Brito, Almeida, Ferreira, & Guisande, 2011). The structural validity of ECCOs subtests have been explained in previous studies (Brito et al., 2011; Martins, Alves, & Almeida, 2016)

The personal and socio-familial variables considered were obtained from children, parents and teachers. School performance was obtained from the respective teachers, using a rating system ranging from 1 to 5 points (1 and 2 = failure; 3–5 = approval), and an overall grade was calculated (average grade).

Procedure

First, it was submitted an authorization request to the Ministry of Education and to the ethics committee of the University. Before administrating the ECCOs, the school principal, parents, and students were made aware of the study purposes, data anonymity and confidentiality, and relevance of participation in a research project of this nature. Formal voluntary consent was given by all of those involved. Students performed the ECCOs subtests in approximately 90 minutes. All instructions in the manual were strictly followed. The scores on ECCOs have been calculated taking the conversion of the raw scores on standardized values considering the children age.

Statistical procedure

In this study we tested a full structural equation model (SEM) using MPlus software (version 7.11) (Muthén & Muthén, 1998–2014). Given the categorical variables in this model the WLSMV estimator was used. Overall model fit was assessed using the following indices: the Comparative Fit Index (CFI; Bentler, 1990) and the Tucker-Lewis index (TLI; Bentler & Bonnet, 1980) with a cutoff value of equal to or greater than .95; the root mean square error of approximation (RMSEA; Steiger, Shapiro, & Browne, 1985) with a cutoff value of equal to or less than .06, values equal to or over .10 are unacceptable (Marôco, 2014). Also, χ² and degrees of freedom are reported. To compare the models we considered the data fit indices CFI and RMSEA. For one model to be considered with less error than another, the RMSEA difference has to be less than .015 (favoring the lower value); at same time one model is better than other when CFI difference is greater than .01 (favoring the higher value) (Chen, 2007; Cheung & Rensvold, 2002). To compare the data obtained in different school grades we use the intervals. A confidence interval (CI) of 90% for the mean direct effects is reported. It should also be noted that the percentages presented in the obtained results are calculated considering the load value squared and then multiplied by 100.

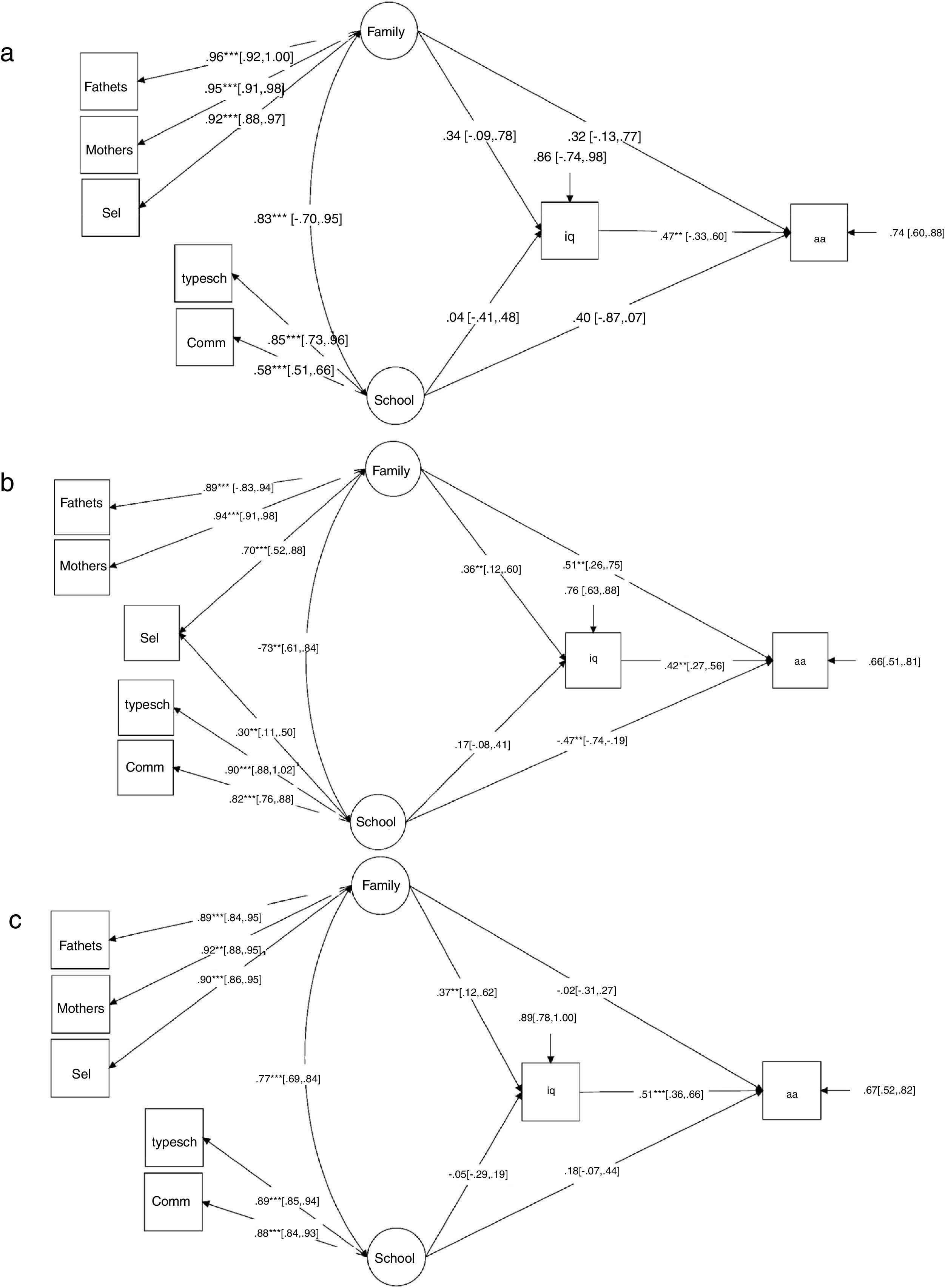
Results

Supported by recent bibliographical entries on the subject, we present the model tested for the different school years (preschool, 2nd year and 4th year of schooling) and for the global sample. The model has two latent variables, family and school. The latent variable family has been estimated considering the father's schooling, the mother's schooling and the family socioeconomic level (low, medium, and high). The latent variable school has been estimated taking the community (rural or urban) and the type of school (public or private). The initial structure of the models was equal in the three years of schooling. However, to achieve a better data fit indices, in the second year the socioeconomic level was directly explained by the two latent variables (family and school). Thus, we obtained a good fit model in the three years of schooling considered, where in preschool: χ²(10) = 15.903, p > .05, RMSEA = .063 with a confidence interval of [.000; .118], CFI = .998 and TLI = .997; in the 2nd year: χ²(9) = 14.632, p > .05, RMSEA = .071 with a confidence interval of [.000; .135], CFI = .998 and TLI = .995; and in the 4th year: χ²(10) = 18.186, p = .05, RMSEA = .078 with a confidence interval of [.000; .135], CFI = .996 and TLI = .992. Given that the models of the three school levels (preschool, 2nd grade and 4th grade) presented good adjustment indices with a similar structure (Fig. 1a–c), we prioritized the analysis of the global results, where we again obtained good fit indexes: χ²(10) = 37.754, p < .001, RMSEA = .083 with a confidence interval of [.056; .112], CFI = .996 and TLI = .993. As can be seen (Fig. 2a), the latent variable family strongly explains the variance of all its observable variables (all the β scores presented are standardized): father's schooling with β = .90, mother's schooling with β = .93, and socioeconomic level with β = .92, which means 81.0%, 86.5% and 84.6%, respectively, of variance is explained. The latent variable school also strongly explains the observable variables community and school type, with β = .75 (56.3% of variance explained) and with β = .88 (77.4% of variance explained), respectively. It is also worth mentioning the strong correlation between the two latent variables, where β = .80 with a confidence interval of [.75, .86]. Although these two variables are strongly correlated, our interest is to observe the causal effect of each on IQ and academic achievement.

Figure 1.

Structural Equation Modeling (SEM): (a) preschool; (b) 2nd year of schooling; (c) 4th year of schooling. Note. Fathers = father's schooling, mothers = mother's schooling, sel = socioeconomic level, typesch = type of school, comm = community, iq = intelligence quotient, aa = academic achievement. *p < .05; **p < .01; ***p < .001.

Figure 2.

Structural Equation Modeling (SEM): (a) global sample; (b) model new. Note. Fathers = father's schooling, mothers = mother's schooling, sel = socioeconomic level, typesch = type of school, comm = community, iq = intelligence quotient, aa = academic achievement. *p < .05; **p < .01; ***p < .001.

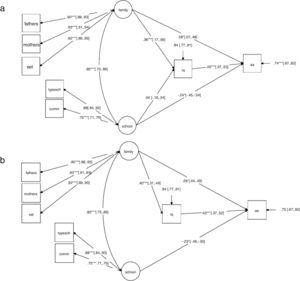
Looking at the data in Fig. 2a, we found that all relationships are statistically significant, except for the causal relationship of the latent school variable in IQ. Given this finding, it was crucial to make a new analysis by eliminating this non-statistically significant relation and again assessing the fit of the model. As the fit of the model was very similar with a confidence interval of 90% (χ²(11) = 35.723, p < .001, RMSEA = .074 with a confidence interval of [.048; .102], CFI = .997 and TLI = .994), with a difference of values ≤.015 of RMSEA (lower value in the last model), we conclude that the latent variable school has no causal impact on IQ, and this new model is now maintained (Fig. 2b). Thus, the variable family has a direct causal relationship with IQ (β = .40, 16% explained variance), but the variable school has no direct causal relation with IQ. However, both latent variables have an equal causal impact on academic achievement, as the confidence intervals overlap (Fig. 2b). The negative sign we see in the causal relationship between the latent variable school and the IQ variable indicates the direction of this relationship; that is, children from the rural community attending public schools obtain better school results. It is also important to mention the higher causal impact of IQ on academic achievement comparing with impact of latent variables family and school. Academic achievement is explained by family, school and IQ in 43.8% of its variance, with a residue of 56.3% (unexplained variance).

In order to finalize the analysis, comparing the statistically significant intervals obtained in the three school years (Fig. 1a–c), values with the same amplitude are obtained, which means they are not different at a 90% confidence interval, suggesting invariance with an increase in schooling.

Discussion and conclusions

The family and school are two fundamental institutions that, at present, share educational functions, contributing to and influencing the development of children. The integration of school and family has aroused the interest of researchers, especially regarding the implications of this involvement for cognitive development and academic achievement (Álvarez et al., 2015; Fernández-Zabala et al., 2016; Oostdam & Hooge, 2013; Regueiro et al., 2015). Their role in cognitive development and academic achievement can be assumed to be complementary, as well as with specific contributions. For example, while some authors argue that schooling perpetuates socio-familial background conditions (Burger, 2010; Magnuson et al., 2004; Sullivan, 2001), others point out that schooling may reduce differences in cognitive performance regardless of socio-familial conditions (Ainscow et al., 2010).

The results obtained in this study point to a significant impact of family on IQ, suggesting a fundamental role in the first years of life as a promoter of cognitive development and school learning (Lugo-Gil & Tamis-LeMonda, 2008; Mistry et al., 2010; Phillipson, 2010; Pomerantz, & Dong, 2006; Raikes et al., 2006). Thus, higher academic qualifications for parents and a higher socioeconomic level appear to be associated with higher IQ for children, corroborating what is reported in the literature (Alves et al., 2016; Bracken & Fischel, 2008; Rindermann et al., 2010).

Regarding the variable school, the results do not confirm its impact on children's IQ. This lack of impact can be explained by the reduced number of years of schooling that children experience, since they attend their first years of schooling (preschool and the first cycle of basic education). So, in these age groups, family seems to be a stronger determining factor than school on cognitive performance. On the other hand, it is also possible to explain this lack of impact in terms of the nature of the variables considered in the description of schools, which are less focused on their pedagogical activity and more on the variables of the surrounding community (urban or rural, public or private).

Regarding academic achievement, family and school play a determinant role, a situation that is also reported in research involving the area (Alves et al., 2016; Eslava et al., 2016; Kloosterman et al., 2011; Lugo-Gil & Tamis-LeMonda, 2008; Mistry et al., 2010). Similar to the impact on children's IQ, parents with higher academic qualifications and higher socioeconomic status are also associated with higher academic achievement of their children.

Another result obtained is that children attending rural schools and public schools present better school classifications. Perhaps children placed in private schools may be subject to higher levels of demand, even because their families have a most favored socio-cultural origin, which may explain their lower school marks (it is important to note that school classifications are not obtained from any national normative parameters). This situation can also be extended to schools in urban areas (compared to rural schools), where we can expect greater academic demands from parents and teachers. Namely in the early school years, academic achievement combines children's learning outcomes and teacher and parent expectations.

In conclusion, this study confirms the important role of family in children's cognitive performance and academic achievement, but school only assumes a significant impact on academic achievement. This lack of school impact on IQ can be explained by the low number of years of schooling for children in present sample, but some methodological limitations must be considered concerning these conclusions. The first concerns the transversal nature of the study, preventing correct analysis of the impact of family and school as a child's schooling progresses. A second limitation is the absence of descriptive variables of the family's educational community and pedagogical practices in the school, which makes it difficult to analyze the results more deeply. Particularly in relation to school, differentiation in terms of community (rural or urban) and status (public or private), as occurs in the study, does not guarantee secure information about the involved teaching and learning processes. Finally, academic achievement, based on an average of the grades attributed by the teachers, assumes some inconsistencies because in these age groups, they do not only reflect the children’ cognitive acquisitions (knowledge, skills and abilities).

Conflict of interest

The authors of this article declare no conflict of interest.

Acknowledgments

This work was supported by the Fundação para a Ciência e Tecnologia, IP (FCT) and the POCH/FSE under Grant SFRH/BD/85110/2012.